Review - Circuits

1.

A length of #8 copper wire, which has a radius of 1.63 mm, carries a steady current of 20 A. The number of electrons per unit volume for copper is 8.47 x 10²⁸ m^-3 and its resistively r= 1.56 x 10^-8 W-m. Find (a) the average current density J, (b) the drift velocity, and (c) the electric field E.

2.

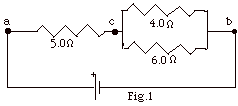
In Fig. 1 below, find V_ab  if the current through the 4.0-ohm resistor is 3.0 A.

3.

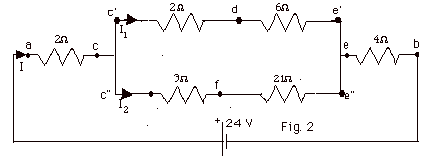
For the circuit of Fig. 2 below, find the equivalent resistance (a) R_c’e’, (b) R_c”e”, (c) R_ce, (d) R_ab.   Find (e) I,  (f) V_ce,  (g) I₁,  and (h) I₂.

4.

Two light bulbs of resistance R₁ and R₂,  respectively, with R₁ > R₂ are connected (a) in series and (b) in parallel. Which bulb is brighter in each case? Assume the resistances of the bulbs remain constant for each wiring.

5.

A battery of emf = 30 V and internal resistance r = 0.5 W  is connected in series with two resistances of 9.5 W and 20 W  (Fig.3 below). Find (a) the current  I  in the circuit, (b) the power dissipated in the external and internal resistances and (c) the power supplied when chemical energy is changed into electrical energy.

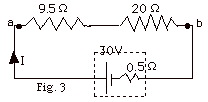
6.

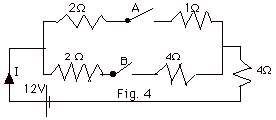
Find the current  I  in the circuit of Fig. 4 below when (a) both switches A and B are closed, (b) only switch A is closed and (c) only switch B is closed.

7.

Two resistors of 10 ohm and 15 ohm in parallel are in series with a 4.0-ohm resistor. The combination is in parallel with a 10-ohm resistor. (a) Draw a diagram for this circuit and (b) find its equivalent resistance.

8.

When a resistor with R = 2.0 ohm is connected to the terminals of a battery, the current through the battery is 6.0 A. When R is replaced by R’ = 7 ohm, the current is 3.0 A.  Find (a) the emf and (b) the internal resistance of the battery.

9.

Find (a) I₁,  (b) I_2,  and (c) I₃  for the circuit of Fig. 5 below.

10.

For an R-C circuit with an initial charge Q_i = 100 µC, resistance R and capacitance C,  the charge decays as a function of a time such that
q(t) = Q_i e^-t/RC.  The time constant is defined as the time for the capacitor to decay until its charge q(t) = Q_i/e.  Find (a) the time constant for the circuit of Figure 6 below and (b) the time when q = 50 µC.
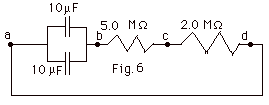