|
|
|
1.
|
(a) Find the magnitude and direction
of the force of +Q on qo at (i) P1 and
(ii) P2 in Fig 1a below. (b) Find the magnitude
and direction of the force of -Q on qo at (i) P1
and (ii) P2 in Fig.1b below. Take Q = 2 x 10-6
C and qo = 10-12 C.
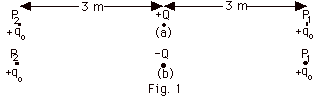
|
|
|
2.
|
Now imagine that all the +qo's
are removed from Fig. 1 above. Find the magnitude and
the direction of the electric field (a) in Fig. 1a and (b)
in Fig. 1b at P1 and P2.
|
|
|
3.
|
(a) Find the electric field at point
P in Fig. 2 below. (b) Repeat for q2 = +1 nC.
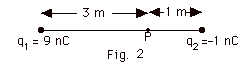
|
|
|
4.
|
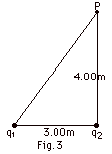
In Fig. 3 below, q1
= +1.00 µC and q2 = -0.0800 µC. Find
the direction and magnitude of the electric field at point
P.
|
|
|
5.
|
Find (a) the electric field, direction
and magnitude, at P (4.00 m, 5.00m) in Fig. 4 below due
to q1 = +5.00 x 10-6 C at (0, 2.00 m),
q2 = -3.00 x 10-6 C at
(4.00 m, 0), and q3 = +1.6 x 10-6 C
at (0, 5.00 m) and (b) the force on q4 = +2.0 x
10-6 C placed at P.
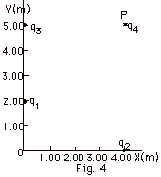
|
|
|
6.
|
Two positive point charges repel each
other. Explain this experimental result in terms of the field
of one charge acting on the other charge.
|
|
|
7.
|
A small positive charge is placed in
the electric field of a fixed positive point charge. Is the
acceleration of the small positive charge constant? Explain
your answer.
|
|
|
8.
|
Two point charges, q1 = +1.0
x 10-6 C and q2 = -4.0 x 10-6 C,
are separated by a distance of 0.10 m. First discuss two of
three points in the figure below where the electric field
cannot be zero and then find the point along the line passing
through the two charges at which the electric field due to
them is zero.
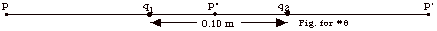
|
|
|
9.
|
(a) If a Gaussian surface has 15 field
lines (the electric flux) leaving it when it surrounds a point
charge of +10.0 µC and 75 field lines entering it when
it surrounds an unknown point charge, what is the amount of
charge on the unknown? (b) If a net number of electric field
lines point out of a Gaussian surface, does that mean there
are no negative charges in the interior?
|
|
|
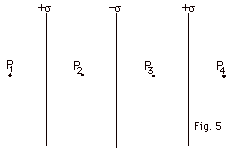
10.
|
Three infinite sheets of charge are parallel
to each other, as is shown in Fig. 5 below. The sheet on the
left has a uniform surface charge density +s,
the one in the middle a uniform surface charge density -s
and the one on the right a uniform surface charge density
of +s. Find the electric
field at (a) P1, (b) P2,
(c) P3, and (d) P4.
|
|
|
11.
|
The number of electric field lines per
unit area crossing a surface at a right angle to the surface
equals the electric field at the surface. Show that the number
of field lines emanating from a point charge +Q is 4pkQ
= Q/eo. Hint:
Surround the charge Q by a hypothetical spherical surface
of radius r and find the product of the electric field due
to Q and the surface area of the sphere.
|
|
|
12.
|
Charge Q is distributed uniformly throughout
a sphere of radius R (Fig. 6 below). Find the electric field
for (a) r ≤ R, (b) r ≥ R. (c) Sketch
a graph of E as a function of r.
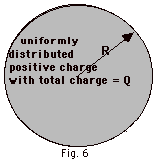
|
|
|
13.
|
A conducting sphere of charge +Q and
radius a is concentric with a conducting, spherical shell
that has a net charge of 0. The shell has inner radius b and
outer radius c. (a) Where are the charges?
Find the electric field for (b) r ≤ a, (c) a ≤
r ≤ b, (d) b ≤ r ≤ c and (e) r ≥ c.
|
|
|
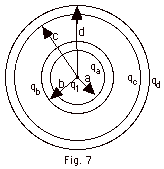
14.
|
A point charge qi = 8.85 µC
is at the center of two concentric conducting shells as shown
in Fig. 7 below. The net charge on the inner shell is -17.70
µC and the net charge on the outer shell is +8.85 µC.
Find (a) the inner charge qa and the outer charge
qb on the inner shell and (b) the inner charge
qc and the outer charge qd on the outer
shell. (c) Sketch a graph of the net flux through a Gaussian
sphere centered on the point charge as a function of the distance
r from the point charge.
|
|
|
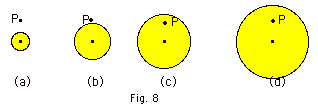
*15.
|
Figure 8 below represents four spheres
of different radii R, but with the same total charge Q distributed
uniformly through the spheres. Point P in the figures is the
same distance from the center of each sphere. (a) Rank the
spheres according to their volume charge density r,
greatest first. (b) Rank the spheres according to the magnitude
of the electric field they produce at point P, greatest first.
*Taken
from Halliday,/Resnick/ Walker, Fundamentals of Physics, Sixth
Edition, Wiley
|
|
|
16.
|
Can two electric field lines intersect?
Explain your answer.
|
|
|
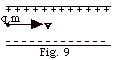
17.
|
A particle of mass m = 1.6 x 10-27
kg and charge q = +3.2 x 10-19 C , moving with
a constant horizontal velocity v = 4.0 x 106 m/s
enters the constant electric field E = 20 x 102 N/C
between the parallel plates of Fig. 9 below. Find (a) the
magnitude and direction of the electric force on the particle
due to E, (b) the acceleration due to the electric field,
(c) the acceleration due to the gravitational force, (d) how
long it takes for the particle to leave the plates if the
length of the plates is 0.80 m and (e) how far it will be
from halfway between the plates as it leaves the plates.
|
|
|
18.
|
A proton of charge +e and mass m is placed
in an electric field E. Find (a) the acceleration of the proton
and (b) the velocity and displacement of the proton in time
t after entering the field. Assume the initial velocity of
the proton is zero.
|
|
|
19.
|
An alpha particle has charge +2e and
a mass that is four times the mass of a proton. If an alpha
particle is placed in the same electric field as the proton
in Problem 18, compare the acceleration, velocity and displacement
of the alpha particle with that of the proton. Again assume
that the initial velocity of the alpha particle is zero.
|
|
|
20.
|
Discuss the possible difference between
the force on a charged particle in a constant electric field
when its initial velocity is zero and when it has an initial
velocity.
|
|
|
21.
|
An electron moves in a circular path
around a long, uniformly charged wire carrying +2.5 nC/m.
What is the speed of the electron?
|
|
|
22.
|
A small sphere with mass m carries charge
q. It hangs from a silk thread that makes an angle Q
with a large, charged nonconducting sheet (Fig. 10 below).
Find the sheet's surface charge density s.
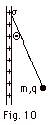
|
|
|
23.
|
Two identical dipoles are placed in a
straight line as shown in Fig. 11a below. Find the direction
of the electric force on each dipole in Fig. 11a. Repeat
for
Fig. 11b below.
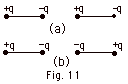
|
|
|
24.
|
An electric dipole consisting of charges
+3.2 x 10-19 C and -3.2 x 10-19 C separated
by 2.0 x 10-9 m is in a field of 5.0 x 105
N/C. Calculate the torque on the dipole when the dipole
moment is (a) parallel and in the same direction as the field
(b) perpendicular to the field and (c) parallel and in the
opposite direction of the field.
|
|
|
25.
|
There are really two types of problems
concerning electric fields (a) given a distribution of charges,
find the electric field due to them and (b) given an electric
field E, find the electric force Fe
on a charge q. Explain two ways of calculating electric fields
and how you find the electric force on a charge q in an electric
field.
|
|