Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 106

Answers - Potential Energy, Potential, and Capacitors

1.
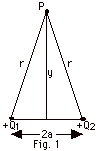
  1. The distances from Q1 and Q2 to P are r = (y2 + a2)1/2.  Since V is a scalar quantity, the potentials add algebraically.

    V = k(Q1/(y2 + a2)1/2 + Q2/(y2 + a2)1/2) = k(Q1 + Q2)/(y2 + a2)1/2.
    Since a is a constant, V is a function only of y.

  2. For y2 >> a2,  V = kQ/y, where Q = Q1 + Q2 which is the same as the potential due to a point charge Q located at the origin.

2.
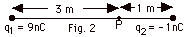
  1. The potential V is a scalar quantity. In this case you may use a minus sign to calculate the potential. For a point charge Q, the potential at a point P a distance r from P is V = (9 x 109 N-m2/C2)Q/r.

    For q1,  V1 = (9 x 109 N-m2/C2)(9 x 10-9 C)/3 m = 27 N-m/C
    = 27 J/C = 27 V.

    For q2,  V2 = (9 x 109 N-m2/C2)(-1 x 10-9 C)/1 m = -9 V.
    V at P = (27 - 9) V = 18 V.

  2. The potential at P is really the potential difference between point P and a very large distance from P. At an infinite distance, the potential due to q1 and q2 is zero (r = a very large number),  V∞ = 0. The work done to bring a charge q3 from a very great distance to P is the increase of the potential energy of the system. The potential difference VP∞ = the potential energy difference divided by q3 = (UP - U∞)/q3.

    Work done = q3VP∞ = q(VP - V∞)= ( 3 x 10-9 C)(18 J/C - 0) = 54 nJ.

  3. For q2 = +1 nC,
    V1 = (9 x 109 N-m2/C2)(9 x 10-9 C)/3 m = 27 N-m/C = 27 J/C = 27 V.
    V2 = (9 x 109 N-m2/C2)(1 x 10-9 C)/1 m = 9 V.
    V at P = (27 + 9).   V = 36 V.
    Now the work done = q3(VP - V∞) = ( 3 x 10-9 C){(36 J/C) - 0} = 108 nJ.

3.
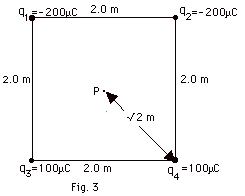
  1. In general, the potential due to a point charge Q at a distance r is kQ/r.
    For q1 = q2 = -200 x 10-6 C, the potential at P is:
    V = 9 x 109 N-m2/C2(-2 x 10-4 C)/√2 m
       = -12.73 x 105 N-m/C
       = -12.73 x 105 J/C
       = -12.73 x 105 V.
    For q3 = q4 = 100 x 10-6 C, the potential at P =
    V = 9 x 109 N-m2/C2(10-4 C)/√2 m = 6.36 x 105 V.
    Due to all the charges,
    V = 2(-12.73 + 6.36)105 V
       = -12.7 x 105 V.


  2. Work to bring q5 = 20 x 10-6 C from infinity =
    q5(VP – V¥) = 20 x 10-6C(-12.7 - 0)105 V = -25.4 J.

4.
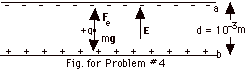
  1. The parallel plate capacitor with its charge and the particle with the forces acting on it are shown in the figure above.  For the particle to be at rest, the net force acting on it must equal zero:  Fnet = ma = m(0).  Taking up to be positive, Fe - mg = 0  or Fe = mg, where the electric force on the particle with charge q in an electric field E is Fe = Eq.  Thus,
    Eq = mg  or
    E = mg/q = (10-3 kg)(9.8 m/s2)/(10-6 C) = 9.8 x 103 N/C.
    The direction of the electric field is up.  Remember the direction of the electric field is that in which a positive charge is urged.

  2. Vab = Ed = 9.8 x 103 N/C (10-3 m) = 9.8 J/C = 9.8 V.

5.



This is a problem in conservation of energy. The potential of a sphere of charge equals the potential of a point charge = kQ/r. When the alpha particle is a long distance away from the gold nucleus initially i, the potential energy of the system Ui is 0.

When the alpha particle touches the gold nucleus, r = 7.0 x 10-15 m = the radius of the gold nucleus.  The potential energy for the "final" position Uf equals:
(9 x 109 N-m2/C2)(79)(2) x (1.6 x 10-19 C)2/(7.0 x 10-15 m)
= 5.2 x 10-12 J.
From conservation of energy,
Ui   +   Ki   =        Uf            +   Kf
 0   +   Ki   = 5.2 x 10-12 J  +    0

6.

Ui   +        Ki       =   Uf  +   Kf   or   Ui - Uf = q(Vi - Vf) = 1/2 mvf2 - 1/2 mvi2
 1.6 x 10-19 C(100 J/C) = 1/2(1.67 x 10-27 kg)vf2 - 0
vf = (2 x 1.6 x 10-17 J/1.67 x 10-27 kg)1/2 = 1.38 x 105 m/s

7.
  1. For the -q and + Q system, the potential energy U = -kqQ/r which remains constant if the distance r of separation of the two charges remains constant.

  2. The electric force does no work because the force is always perpendicular to the displacement of the negative charge when it moves in a circular path around the positive charge. Thus, there is no potential energy difference between points along the circular path. The circle is an equipotential path.

8.

 Ua - Ub = q(Va - Vb)
  1. For the proton,
    Ua - Ub = 4.8 x 10-19 J = (1.6 x 10-19 C)(Va - Vb)
    (Va - Vb) = Vab = (4.8 x 10-19 J)/(1.6 x 10-19 C) = 3.0 V.

  2. For the alpha particle,
    Ua - Ub = q(Vab) = (3.2 x 10-19 C)(3.0 V) = 9.6 x 10-19 J.

9.



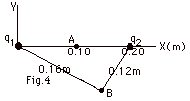
(a) VA = 9.0 x 109 N-m2/C2[80/0.10 - 60/0.10]10-9 C/m = 1.8 x 103 V

(b) VB = 9.0 x 109 N-m2/C2[80/0.16 - 60/0.12]10-9 C/m = 0

(c) WBright arrowA = q(VA - VB) = (10 x 10-6 C)(1.8 x 103 V - 0) = 0.018 J

10.

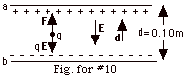
  1. If the kinetic energy of the object with charge q is to remain constant, the net force acting on the object must equal zero. For this to be true,
    Fnet = F + qE = 0,
    where F is the force applied by the outside agent. The work done in carrying the object from b to a without increasing its kinetic energy producing a change in potential energy difference = F . d = -qE . d.

  2. Ua - Ub = -qE . d = -qEd cos 180o = -qEd(-1) = qEd
    =10-6C(104N/C)(0.10m) = 10-3 J.

  3. qVab = Ua - Ub = qEd.
    Vab = Ed = (104 N/C)(0.10 m) = 103 V.

11.
  1. WBright arrowA = F . d, where F is applied by an external agent and for only an increase in potential energy Fnet = F + qE = 0  or  F = -qE  and
    WBright arrowA = -qE . s = -q(Exsx + Eysy) =
    -10-6 C[(6)(-4) + (-8)(4)]104 N-m/C = 0.56 J.
    For a conservative force, the work done is independent of the path.

    As you go from B to C, - the work done by the electric field =
    -10-6 C(6 x 104 N/C)(-4 m) = 0.24 J.

    From C to A, -work done by the electric field =
    -10-6 C(-8 x 104 N/C)(4 m) = 0.32 J.

    Again the total work done = (0.24 + 0.32)J = 0.56 J.

  2. UA - UB = -qE . s = 0.56 J.

  3. qVAB = UA - UB. VAB = (UA - UB)/q = 0.56 J/10-6 C = 56 x 104 V.

12.



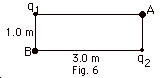
  1. VB = kq1/1.0 m + kq2/3.0 m
         = 9 x 109 N-m2/C2[(-4.0 x 10-6 C/1.0 m) +(2.0 x 10-6 C/3.0 m)]
         = 3 x 103[-12 + 2] J/C = -30 x 103 V

  2. VA = kq1/3.0 m + kq2/1.0 m
        = 9 x 109 N-m2/C2[(-4 x 10-6 C)/3.0 m) + (2.0 x 10-6 C/1.0 m)]
        = 3 x 103[-4 + 6] J/C = 6 x 103 V

  3. Since no work is done to change the kinetic energy of the particle, the work done to move q3 from B to A =
    UA - UB = q3(VA - VB) =
    (3.0 x 10-6 C)[6 - (-30)] 103 J/C = 0.108 J.

  4. The electric force is a conservative force so the work done is independent of the path.

13.
  1. U = -ke2/r

  2. Fnet = ma
    ke2/r2 = mv2/r  or  mv2 = ke2/r

  3. E = U + K = -ke2/r + 1/2 mv2 = -ke2/r + 1/2 ke2/r = -ke2/2r
    L = mvr

14.
  1. U = -ke2/2r

  2. At distance 2r, the electron is momentarily at rest and K = 0.
    E = U + K = -ke2/2r + 0.

  3. The total energy is the same for motion in a circle of radius r and for the skinny ellipse approximated by motion back and forth in Fig. 7c above. For Fig. 7c,   the angular momentum L = 0.  Note: The quantum number   "represents" angular momentum. For the orbital model, the total energy would be the same for both, but the quantum number    would be different. For more complex atoms, there is penetration of the "inner core" for low angular momentum and the total energy depends on the quantum number  .

15.
  1. When capacitors are wired in parallel, the equivalent capacitance
    C = C1 + C2 + C3.
    But, C1 = C2 = C3 = e0A/d,  so C = 3e0A/d = e0A/(d/3).
    The plate spacing for the single capacitor must be d/3.

  2. When capacitors are wired in series, the reciprocal of the equivalent capacitance 1/C = 1/C1 + 1/C2 + 1/C3 = 3/C1 and C = C1/3 = (e0A/d)/3 = e0A/3d, so the plate spacing for the single capacitor must be 3d.

16.

 For any capacitance, including C1,  C1 = Q/(Vab)i, where Q is the charge on the capacitor when (Vab)i is the potential difference across it. When the two capacitors are wired in parallel, the potential difference across each is the same = (Vab)f = q1/C1 = q2/C2, where q1 and q2 are the charges on C1 and C2 with potential difference (Vab)f. Also from conservation of charge q1 + q2 = Q or q2 = Q - q1 = C1 (Vab)i - C1 (Vab)f = C1{(Vab)i - (Vab)f}. But q2 also equals C2 (Vab)f. Thus C1{(Vab)i - (Vab)f} = C2(Vab)f or since C1 = 4pe0R1 and C2 = 4pe0R2, 4pe0R1 {(Vab)i - (Vab)f} = 4pe0R2(Vab)f . Thus R2 = R1{(Vab)i - (Vab)f}/(Vab)f.

17.

 The capacitance of a parallel plate capacitor in a vacuum C = eoA/d, where A is the area of the plates and d is the distance between them.
  1. When the plate separation is doubled:

    1. The capacitance C is halved since the capacitance is inversely proportional to the distance d.

    2. C = Q/Vab or Q = CVab. When C is halved and Vab remains the same (the battery is still across the capacitor), the charge Q is halved.

    3. E = s/eo. When Q is halved, the charge per unit area s is halved and E must be halved. Also E = Vab/d so when d is doubled E is halved.

  2. When a dielectric with k= 2 is inserted:

    1. The capacitance = k eoA/dso the capacitance is doubled.

    2. Q = CVab. When the capacitance doubles, with constant Vab, the charge doubles.

    3. E = s/keo. When Q doubles, s doubles, but s/k remains the same because k= 2 and E remains the same. Also E = Vab/d so E is the same because Vab and d remain the same.

18.

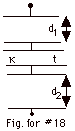
We may view the capacitor as three capacitors in series, shown in Fig. for #18 above.

1/Ceq = 1/C1 + 1/C2 + 1/C3 = d1/Ae0 + t/Ake0 + d2/Ae0.
Ceq = Aeo/(d1 + t/k + d2).

19.


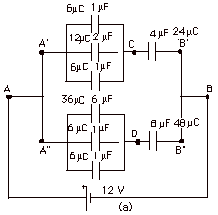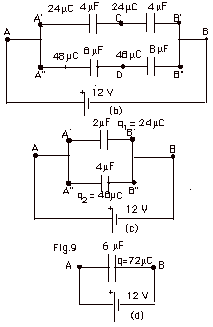To find the equivalent capacitance of Fig. 9 we start with the original circuit.

The capacitors between A’ and C are in parallel so CA’C = (1 + 2 + 1)µF = 4 µF. The capacitors between A’ and D are in parallel so CA’D = (6 + 1 + 1)µF = 8 µF, as shown in Fig. 9 b above.

The capacitors between A’ and B’ are in series.
1/CA’B’ = 1/4 µF + 1/4 µF = 1/2 µF  and  CA’B = 2 µF, as shown in Fig 9c.

The capacitors from A” to B” are in series so 1/CA”B’” = 1/8 µF + 1/8 µF =
1/4 µF  and  CA’B = 4 µF, as shown in Fig 9c.

The capacitors between A and B (Fig. 9c) are in parallel and CAB = 6 µF (Fig. 9d above).

Now work backwards to find the charge.
qAB = CAB VAB = 6 x 10-6 (C/v) 12 V = 72 x 10-6 C = 72 µC.
Up to Fig. 9c,  qA’B’ = CA’B’ VA’B ’ = 2 x 10-6 (C/V) 12 V = 24 x 10-6 C = 24 µC. VqA”B” = CA”B” VA”B” = 4 x 10-6 (C/V) 12 V = 48 x 10-6 C = 48 µC.
Notice qA’B’ + qA”B” = (24 + 48)µC = 72 µC. The two capacitors from A’ to B’ are in series so each has charge = 24µC and the two capacitors from A” to B” are in series so each has charge = 48µC. VA’C = VDB’ = 24 x 10- 6 C/4 x10-6 C/V = 6 V.


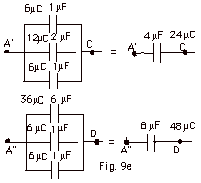In Fig. 9e above, from A’ to C, the charge on the two 1µF capacitors is

q1µF = 1 x 10-6 C/V(6V) = 6 x 10-6 C = 6µC,
and the charge on the 2µF capacitor is
q2µF = 2 x 10-6 C/V(6V) = 12 x 10-6 C = 12µC.
In Fig. 9e, the charge on the 6µF capacitor is
q6µF = 6 x 10-6 C/V(6V) = 36 x 10-6 C = 36µC,
and the charge on the two 1µF capacitors is
q1µF = 1 x 10-6 C/V(6V) = 6 x 10-6 C = 6µC.
Check Fig. 9e to see how the charges on the parallel combinations add up nicely to be equal to the charge of their equivalent capacitances.

20.



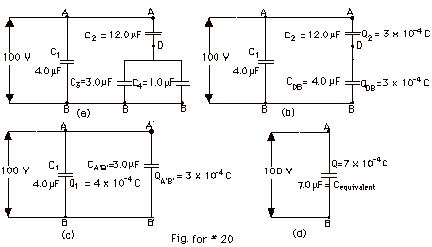
(a) We find the equivalent capacitance by starting with the parallel combination between D and B.

Capacitors in parallel add.  CDB = C3 + C4= (3.0 + 1.0)µF = 4.0 µF  (Fig. 20b).

CDB and C2 are in series. The reciprocal of the equivalent capacitance equals the sum of the reciprocals of the capacitors in series.

1/CA’B’ = 1/C2 + 1/CDB = 1/12 µF + 1/4.0 µF = (1 + 3)/12 µF  or
CA’B’ = 3.0 µF  (Fig. 20c).

Then C1 and CA’B’ are in parallel.  Cequivalent = (4.0 + 3.0) µF = 7.0µF.
(Fig. 20d)


(b) and (c) Now to find the charges and potential differences we work backwards .

For Fig. 20d,
VAB = 100V = Qequivalent/Cequivalent = Qequivalent/7.0 x 10-6 C/V.
Qequivalent = 7.0 x 10-4 C.

For Fig. 20c,
VAB = 100V = Q1/C1 = Q1/4.0 x 10-6 C/V.
Q1 = 4.0 x 10-4 C.
QA’B’ = 100 V(CA’B’) = 100 V (3.0 x 10-6 C/V) = 3.0 x 10-4 C.
Notice Q1 + QA’B’ = 7.0 x 10-4 C.

For Fig. 20b, C2 and CDB are in series and have the same charge.
Q2 = QDB = QA’B’ = 3.0 x 10-4 C.
VAD = Q2/C2 = 3.0 x 10-4 C/12 x 10-6 C/V = 25 V.
VDB = QDB/CDB = 3.0 x 10-4 C/4 x 10-6 C/V = 75 V.
Notice VAD + VDB = 100 V.
Q3 = C3 VDB = 3.0 x 10-6 C/V (75 V) = 2.25 x 10-4 C
Q4 = C4 VDB = 1.0 x 10-6 C/V (75 V) = 0.75 x 10-4 C.
Notice that Q3 + Q4 = 3.0 x 10-4 C = Q2.


(d) Energy stored in a capacitor = U = 1/2 QV = 1/2 Q2/C.
U1 = 1/2 Q12/C1 = 1/2(4.0 x 10-4 C)2/(4.0 x 10-6 C/V) = 2.0 x 10-2 J.
U2 = 1/2 Q22/C2 = 1/2(3.0 x 10-4 C)2/(12.0 x 10-6 C/V) = 0.375 x 10-2 J.
U3 = 1/2 Q32/C3 = 1/2(2.25 x 10-4 C)2/(3.0 x 10-6 C/V) = 0.843 x 10-2 J.
U4 = 1/2 Q42/C4 = 1/2(0.75 x 10-4 C)2/(1.0 x 10-6 C/V) = 0.281 x 10-2 J.
U1 + U2 + U3 + U4 = 3.5 x 10-2 J.
Uequivalent = 1/2 (Qequivalent)2/Cequivalent
                    = 1/2(7 x 10-4 C)2/(7 x 10-6 C/V) = 3.5 x 10-2 J.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
August 8, 2002
April 23, 2003