Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 107

Outline - Waves
  1. Descriptive Terms

    1. Period T equals the time for one complete oscillation of the source that produces the wave.

    2. Frequency f equals the number of oscillations per second.

    3. Wavelength l equals the distance moved by the wave in one period or in one oscillation.

      l x f = v

      (distance/oscillation) x ( number of oscillations/s) = (distance/s)

    4. Velocity v equals distance moved by the wave per second.


  2. Equation of motion for a traveling wave

    1. The displacement y of a point on a traveling wave depends on the distance x from the source and the time t.  y is a function of x and t.

    2. Each point on the wave vibrates with simple harmonic motion of the same amplitude and frequency, but there is a phase difference between the motion of a point at x and a point at x’ ≠ x  if  x’ ≠ (x ± nl) where n = 0, 1, 2, . . . .  Points separated by l vibrate in phase; points separated by l/2 vibrate 180o out of phase.

    3. y(x,t) = A sin (2px/l ± 2pt/T), where the - sign is used for a wave traveling to the right and the + sign for a wave traveling to the left. If the wave is traveling to the right, you want the function at x > 0 to be equal to the function at x = 0 and t = 0. To insure this you subtract vt and then f(x - vt) = f(0). If the wave is traveling to the left then the x is getting smaller and you have to add vt.

    4. Letting k = 2p/l and w = 2p/T = 2pf, we may write y(x,t) = A sin (kx ± wt).

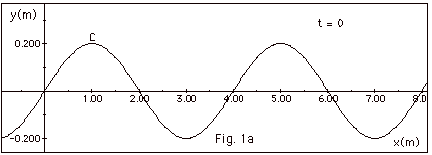
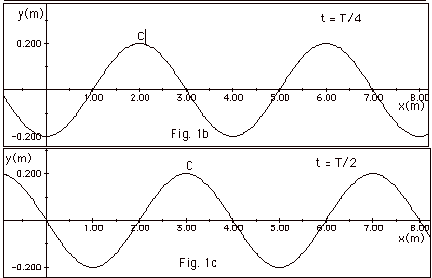
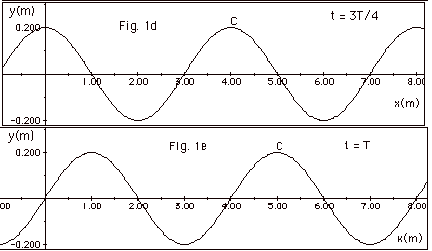
    5. Fig. 1a below shows a travelling wave with l = 4 m at t = 0. A crest C appears at x = 1 m. The following figures show the shape of the wave as it travels to the right. Since a wave travels one-wavelength l in one period T, it travels l/4 in time T/4. In Fig. 1b below, at time t = T/4 the crest C has moved from x = 1.0 m to x = 2.0 m a distance of 1 m = l/4.  At t = T/2, t = 3T/4, and T, the crest has moved l/2, 3l/4, and l, respectively.







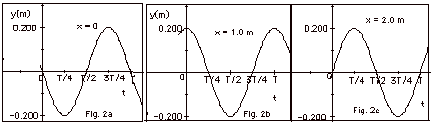
    6. In Fig. 2 below, I have plotted y as a function of time for three values of x. If you look back at Fig. 1 above and concentrate on the point at x = 0 and find the value of y for t = 0, T/4, T/2, 3T/4, and T, you will see that a plot of y as a function of t looks like Fig. 2a below. Now concentrate on the point at x = 1.0 m and you will find that a plot of y as a function of t looks like Fig. 2b below. A plot of y as a function of t for x = 2.0 m is shown in Fig. 2c below. Notice that all points on the wave vibrate up and down with the same amplitude and frequency, but they are not in phase. If you look at Fig. 2a and Fig. 2c below, you find the particles are 180o out of phase.




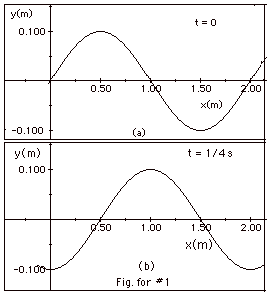
    7. Sample problem 1. For the wave shown in Fig. for #1a below, the displacement of the wave as a function of x is shown for t = 0. In Fig. for #1b below, y is shown as a function of x for t = 1/4 s. This is the first time the wave looks this way after t = 0. Find (a) the amplitude of the wave, (b) the wavelength of the wave, (c) the period of the wave, (d) the frequency of the wave, (e) the velocity of the wave, and (f) the direction in which the wave is moving. Explain your answer to (f).



    8. Sample Problem 2. Given y(x,t) = 4 cm sin (p m-1 x - 4p s-1 t) find (a) the amplitude , (b) the wavelength, (c) the period, (d) the frequency (e) the velocity of the wave and (f) the direction in which it is moving.

    9. More practice problems in 107 Problem Set for Wave Motion: 1-9, 17.


  3. Standing Wave

    1. A standing wave is formed by combining a wave to the right and to the left. For a standing wave,

      y(x,t) = y1(x,t) + y2(x,t)
      y(x,t) = A sin (2px/l - 2pft) + A sin (2px/l + 2pft)
               = A[sin 2px/l cos 2pft - cos 2px/l sin 2pft}
                    + A[sin 2px/l cos 2pft + cos 2px/l sin 2pft}
               = 2A sin 2px/l cos 2pft = (2A sin 2px/l) cos 2pft

    2. This reminds us of the equation for simple harmonic motion: y(x,t) = (Amplitude) cos 2pft or y(x,t) = (Amplitude) cos wt

    3. For a standing wave, all points on the wave do not have the same amplitude.

      1. In fact, when sin 2px/l = 0, the displacement of the rope at those points never have any displacement. These points are called nodal points and occur when 2px/l = 0, p, 2p, 3p, 4p, etc.  A neat way to write this is to say you get a nodal point when 2px/l = mp, where m = 0, 1, 2, 3, 4. . .  or x = mpl/2p or x = ml/2. (A historical note here. This was the first time that a quantity took on integral values or discrete values. In other words, the values were not continuous. This is a foundation of quantum mechanics.) The distance between nodal points = Dx = (m + 1)l/2 - ml/2 = l/2.

      2. Positions of maxima with amplitude 2A, where A is the amplitude of a single wave, are called antinodes. They occur for sin 2px/l = 1 or 2px/l = (m + 1/2)l or x = (m + 1/2)l/2. The distance between antinodes is again l/2.

      3. All points, except the nodal points, on the standing wave vibrate with simple harmonic motion, but the amplitudes vary from zero at a nodal point to a maximum at an antinodal point. The entire string on which a standing wave exists has zero displacement twice each period.

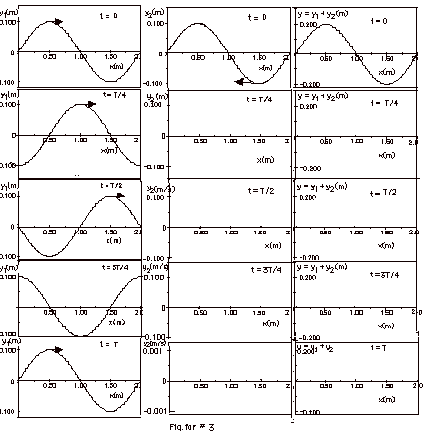
    4. Sample Problem 3. Below, I have drawn y1(x,t), y2(x,t), and y(x,t) = y1(x,t) + y2(x,t) for t = 0. You find y(x,t) by adding the displacements of y1(x,t) and y2(x,t) for a given time t. I have also drawn y1(x,t) for t = T/4, t = T/2, t = 3T/4, and T. Notice that the crest at x = 0.50 m for y1(x,t) at t = 0 moves to x = 1.0 m at t = T/4. In other words, the wave moves l/4 in time T/4. You are to complete the figure by drawing y2(x,t) and y(x,t) for t = T/4, t = T/2, and T = T. How do the y(x,t) compare at time t = 0 and t = T? Is this what you would have expected? What are the distances between nodes in the y vs x curves? What are the distances between antinodes in the y vs x curves?




    5. More practice problems in 107 Problem Set for Wave Motion: 10-16, 22, 23.


  4. Solution to Sample Problems 1, 2, and 3 above

    1. From Fig. for #1 below, (a) the amplitude of the wave is 0.100 m. (b) the wavelength = 2.00 m. (c) In Fig. b, the crest has moved 0.50 m to the right in 1/4 s. Since 0.50 m = l/4, the time elapsed = T/4. T = 1.0 s. (d) The frequency f = 1/T = 1.0 s-1. (e) lf = v = (2.00 m)(1 s-1) = 200 cm/s.
      (f) Since the crest moves to the right, the wave is moving to the right.




    2. Given
                y(x,t) = 4 cm sin (p m-1 x - 4p s-1 t)

      Compare with
                y(x,t) = A sin (2px/ l ± 2pt/T)

      (a) A = 4 cm,
      (b) 2p/ l = p m-1 or l = 2 m
      (c) 2p/T = 4p s-1 or T = 0.5 s
      (d) f = 1/T = 2 s-1 s
      (e) v = lf = (2 m)(2 s-1) = 4 m/s
      (f) The wave travels to the right because f(x,t) = f(x –vt)


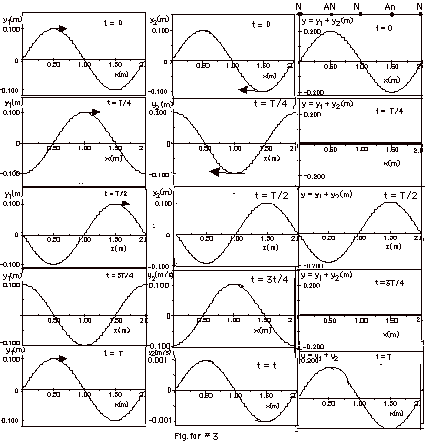
    3. As illustrated in the figure below:
      equation of wave to the right is  y1(x,t) = 0.10 m sin (p m-1x - 2pft);
      equation of wave to the left is  y2(x,t) = 0.10 m sin (p m-1x + 2pft);
      equation of the standing wave is  y(x,t) = 0.20 m sin p m-1x cos 2pft.

      Nodal points are 1.00 m apart = l/2 = distance between antinodal points. After one period T,  the waves look the same as they did for  t = 0.





  5. Boundary Conditions

    1. For a fixed end (rope tied down) at x = 0

      1. At  x = 0,  y = 0

      2. At fixed end, a standing wave must have a node.

    2. For a free end at  x = L

      1. At  x = L,  dy/dx = 0

      2. At free end, a standing wave must have an antinode.

    3. Rope with fixed ends at  x = 0  and  x = L  (Fig. 4 below)




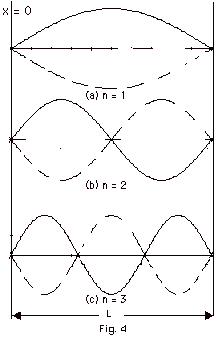
      1. To make  y = 0  at  x = 0,  choose y(x,t) = A sin kx cos wt.

      2. To make  y = 0  at  x = L,  set sin kL = 0  or
        kL = np  and  k = 2p/l = np/L  or  l =2L/n.

      3. f = v/l= nv/2L

      4. For Fig. 4a above,  n = 1,  l1 = 2L ,  f1 = v/2L
        (fundamental or first harmonic)

      5. For Fig. 4b above,  n = 2,  l2 = L ,  f2 = v/L
        (first overtone or second harmonic)

      6. For Fig. 4c above,  n = 3,  l3 = 2L/3,  f3 = 3v/2L
        (second overtone or third harmonic)


    4. Rope with fixed end at  x = 0  and free end at  x = L  (Fig. 5 below)




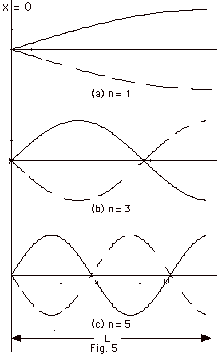
      1. To make  y = 0  at  x = 0,  choose y(x,t) = A sin kx cos wt.

      2. dy/dx = Ak cos kx cos wt

      3. To make dy/dx = 0  at  x = L,
        cos kx = 0  and
        kL = np/2 where n = 1, 3, 5, . . .
        k = 2p/l = np/2L
        l= 4L/n   and   f = nv/4L.

      4. For Fig. 5a above,  n = 1,  l= 4L,  f = v/4L
        (fundamental or first harmonic)

      5. For Fig. 5b above,  n = 3,  l= 4L/3,  f = 3v/4L
        (third harmonic)

      6. For Fig. 5c above,  n = 5,  l= 4L/5,  f = 5v/4L
        (fifth harmonic)


  6.   Reflection of Waves from a Fixed End



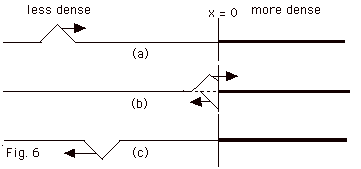
    1. Boundary Condition: y = 0  at  x = 0  at all times t.

    2. Initial conditions:  At time  t = 0,  wave pulse has shape shown in
      Fig. 6a above and is moving to the right.

    3. To find what happens to the pulse when it hits the wall,

      1. Observe reflected pulse in string.

      2. Superposition of incident pulse and reflected pulse with 180o phase change gives  y = 0  at  x = 0  (Fig. 6b above).


  7. Reflection at a Free End



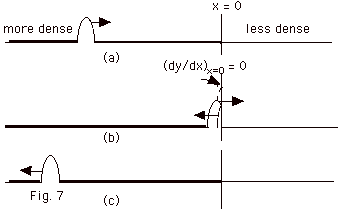
    1. Boundary conditions:  dy/dx = 0  at  x = 0  for all times t.

    2. Initial condition:  At time  t = 0,  wave pulse has shape shown in
      Fig. 7a above and is moving to the right.

    3. To find what happens to pulse when it goes from more dense to less dense medium,

      1. Observe reflected pulse with string.

      2. Superposition of incident pulse and reflected pulse with no change of phase gives dy/dx = 0 at x = 0.  In other words, the displacement of the incident wave plus the displacement of the reflected wave gives a maximum displacement with the slope of the curve equal to zero at  x = 0  (Fig. 7b above).

    4. Practice problems in 107 Problem Set for Wave Motion: 24, 25.


  8.   Doppler Effect

    1. Observed change in the frequency f of the source when the observer or the source is in motion.

    2. Motion of observer

      1. Imagine the source is at rest sending out spherical waves to the observer.  The waves will travel with a velocity v and the crests will be spaced l apart.  If the observer is at rest, she will receive f crests each second because the frequency  f  is number of crests or wavelengths received each second.

      2. If the observer is moving with a speed vobs,  in time t she will move a distance vobs times the time t.  During one second, the observer moves vobs(1 s). The number of crests she receives in that 1 s is vobs/l. The total number of wavelengths the observer receives in one second is
        f' = f + vobs/l      or factoring out  f  gives
        f" = f(1 + vobs/fl)         Equation 1


      3. If the observer moves toward the source, she hears a higher frequency  f  than the frequency f emitted by the source.

        By definition, fl = v,  where v is the velocity of the sound wave. Substituting  fl = v  into Eq. 1 gives
        f' = f(1 + vobs/v)  or
        f' = f(v + vobs)/v          Equation 2
        If the observer is moving away from the source, she receives
        vobs/l fewer per second.  For this case,
        f' = f(v - vobs)/v         Equation 3


      4. We can combine Eq. 2 and Eq. 3 as
        f' = f(v ± vobs)/v             Equation 4
        where the plus sign goes with the motion of the observer moving toward the source and the minus sign with motion of the observer away from the source. You can decide on the sign by remembering the frequency detected by the observer is higher if she is moving toward the source.


    3. Motion of the source



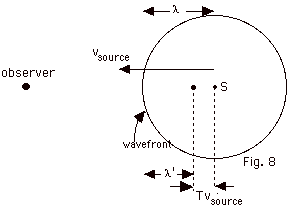
      1. If the source moves toward the observer, the distance between the crests gets shortened on the side approaching the observer.  If the velocity of the source is vsource in time T,  the period of the wave, the source moves a distance of vsource T and the wavelength is shortened to  l’ = l - vsource T  (Fig. 8 above). The observed frequency f’ becomes v/l’, where v is the velocity of the wave. The observed frequency f’ is larger than the emitted frequency f.  Then,
        f’ = v/(l - vsourceT),  or since  1/T = f,
        f' = v/(l - vsource/f)                   Equation 5
        Multiplying the numerator and denominator of Eq. 5 by f yields
        f' = vf/(lf - vsource)               Equation 6
        Recognizing that lf = v = velocity of wave,  Eq. 6 becomes
        f' = f [ v/(v – vsource)]             Equation 7

      2. If the source moves away from the observer,  l’ = l+ vsource T and the observed frequency  f’ = v/l’  is smaller than the emitted frequency f.   Now
        f' = f [ v/(v + vsource)]              Equation 8


      3. We can combine Eq. 7 and Eq. 8 by writing
        f' = f [v/(v± vsource)              Equation 9
        where the minus sign goes with the source moving toward the observer and the plus sign with the source moving away from the observer.


    4. Motion of Observer and/or Source

      1. We can combine Eq. 4 and Eq. 9 to give
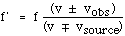                 Equation 10

      2. If the source is not moving, we set  vsource = 0  and proceed as we did with only the observer is moving.

        If the observer is not moving, we set  vobs = 0  and proceed as we did with only the source moving.

        If both the source and observer are moving, we put in both speeds and choose the sign as if only one or the other were moving.


    5.   Practice problems in 107 Problem Set for Wave Motion: 26-28.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
October 8, 2002
April 22, 2003