Solution Set - Geometric Optics

Phyllis Fleming Physics

Physics 108

Answers - Geometric Optics

Note that I have used:

s_o = object distance, s_i = image distance,
Q_i for the incident ray, Q_r for the reflected ray, Q_t for the transmitted ray.

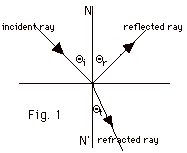
1.	The angle of incidence Q_i is the angle between the incident ray and the normal N. The angle of reflection Q_r is the angle between the reflected ray and the normal N. The angle of refraction (transmission) Q_t is the angle between the refracted ray and the normal N’.

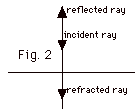
2.	When a light ray hits a surface normally, the angle between the incident ray and the normal is 0, so Q_i = 0. Since the angle of reflection equals the angle of incidence, Q_r= 0. By Snell's law, n_i sin Q_i = n_t sin Q_t. For any value of n_i and n_t, if Q_i= 0, Q_t = 0.

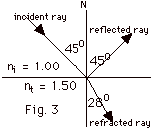
The angle of incidence, the angle between the incident ray and the normal N is given as 45^o. Since the angle of reflection equals the angle of incidence, the angle of reflection is 45^o.
By Snell's law
n_i sin Q_i = n_t sin Q_t1.00 sin 45^o = 0.707 = 1.50 sin Q_t.
sin Q_t = 0.707/1.50 = 0.471. Q_t = 28^o

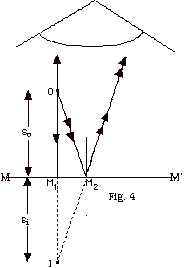
Ray 1, designated by one arrow, hits the mirror normally and is reflected back on itself, as shown in Fig. 4.

Ray 2, designated by two arrows hits the mirror at some angle of incident greater than zero and is reflected back at the same angle. If you dash the reflected rays behind the mirror, you see that they meet at a point that I have labeled I for image.

The big eye seen at the top of the figure sees the two reflected rays as though they have originated at the image. In other words, if you sight along the two reflected rays they appear to have come from the image. This image is called a virtual image because the rays do not actually meet at that point.

The distance of the object O from the mirror, s_o, is called the object distance and the distance of the image I from the mirror, s_i , is called the image distance. If you measure them or use geometry to calculate the distance s_i, you find that s_i equals s_o for a plane mirror.

By geometry,

M₁OM₂ = Q_i (alternate interior angles),
OIM₂ = Q_r (two parallel lines crossed by a line),

and the angle of incidence = the angle of reflection.
Triangles OM₁M₂ and IM₁M₂ are congruent (all angles are equal and they have a common side).
Thus, s_o= s_i.

Using Snell's law to find the angle of refraction, 1.00 sin 37^o= 1.50 sin Q_t.
Q_t = 23.6^o.

The angle of transmission and the angle of incidence on the interface between the glass and the air are equal (a line cut by two parallel lines). Since Q_i= 23.6^oat the glass-air interface = Q_t at the initial air-glass interface, the angle of refraction at the glass-air interface is 37^o from Snell's law.

I have dashed the incident ray to show you that for a parallel plate, the refracted beam is parallel to the incident beam. This tells you that for a very thin parallel plate, the incident beam passes through without any deviation, as with a thin lens.

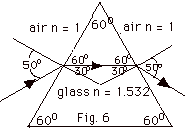
Using Snell's law,

n_i sin Q_i = n_tsin Q_t.

At first interface,

1(sin 50^o) = 1.532 sin Q_t
sin Q_t = 0.766/1.532 = 0.500. Q_t = 30^o.

At the second interface, as the light ray is going from the glass back into the air, Q’_i= 30^o. Now Q’_t= 50^o. The path of the ray is shown in Fig. 6 above.

For 1 sin 50^o= n’ sin Q_t, with n’ less than 1.532, Q_t’would be greater than 30^o. The light for l’ would be bent less toward the normal. When the ray goes from the prism back into the air, n’ sin Q'_i = 1 (sin Q’_t) and the light ray would be bent less away from the normal than the light for l. The overall effect is that the light of wavelength l’ has a smaller deflection from the original direction of the incident beam than light of wavelength l.

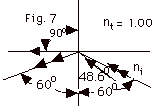
The angle of refraction Q_t= 90^o.
n_i sin Q_i = n_t sin Q_t n_i sin 48.6^o = 1.00 (sin 90^o)
n_i= 1/0.750 = 4/3
For Q_i = 60^o, 4/3 sin 60^o = (1.00) sin Q_t.
sin Q_t = 4/3 (0.866) = 1.15, which is not possible because the maximum value of a sine is 1. What happens is that the ray is totally internally reflected as shown in Fig. 7 above with the rays with two arrows.

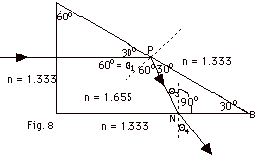
From the geometry of the prism, we see that Q₁ = 60^oand Q₃ = 30^o.
(In triangle PBN, there are 180^o = (30^o+ 30^o+ 90^o+ Q₃).
From Snell's law,
1.655 sin Q₃ = 1.333 sin Q₄
(1.655)(0.5000) = 1.333 sin Q₄ Q₄ = 38.4^o.
Total internal reflection occurs when 1.655 sin Q₁ > n_mixsin 90^o.
Total internal reflection ceases when 1.655 sin 60^o = n_mix sin 90^o = (n_mix)(1). n_mix= 1.655 (0.866) = 1.433.

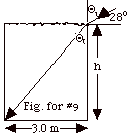
9.	n_isin Q₁ = n_t sin Q_t. Q_i= 90^o- 28^o= 62^o. 1.00 sin 62^o = 1.33 sinQ_t. sinQ_t = 1.00(0.883)/1.33 = 0.664. Q_t= 41.6^o. tan Q_t = 0.888 = 3.0 m/h. h = 3.38 m.

10.

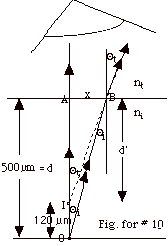
Ray 1 (indicated with one arrow in Fig. for #10) from the scratch at O hits the surface of the slip normally at an angle of incidence of 0^o and continues into the air with no refraction.

Ray 2, (indicated with two arrows) is incident upon the surface of the slip at angle Q_i and transmitted at angle Q_t. The camera or an eye sees these two rays as though they had diverged from the image I.

From the geometry of the figure, we see that tan Q_t = x/d’ and tan Q_i = x/d.

From Snell's law,

n_i sin Q_i = n_tsin Q_t.

For sin Qapproximately equal to tan Q, Snell's law becomes

n_i tan Q_i = n_ttan Q_t.

Or, since tan Q_t = x/d’ and tan Q_i = x/d, n_i x/d = n_t x/d’.
Thus, n_i/n_t = d/d’.

Putting in n_t =1.00, d = 500 µm, and d’ = 500 µm - 120 µm = 380 µm,
n_i/1 = 500 µm/380 µm = 1.32.

11.

n_i sin Q_i= n_t sin Q_t. For the ray incident at the top of the semicircle (Fig. 9),
n_i= 1, n_t= 4/3, and Q_i= 53^o.

From Snell's law,

(1) sin 53^o= 4/3 sin Q_t or
(1)(4/5) = 4/3 sin Q_t,
sin Q_t = 3/5 and Q_t= 37^o.

The transmitted ray travels along a radius, hits the interface between "water and air" normally, and experiences no refraction as it goes into the air.

12.

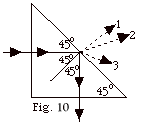
From the geometry of Fig. 10 above, the angle of incidence at the interface between the prism and air is 45^o. For a minimum index of refraction n_pof the prism, n_psin 45^o = n_air sin 90^o or n_p= 1/sin 45^o= 1.41.
If the index of refraction is too small, the refracted ray will be #3 since it bends away from the normal. Ray 1 is the normal and Ray 2 bends toward the normal.

13.

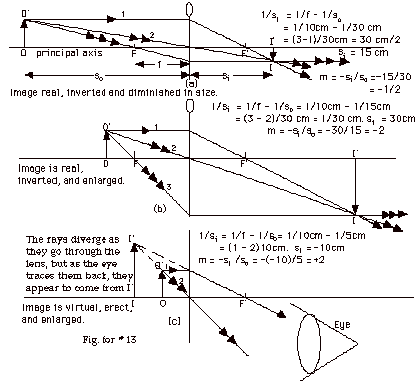
1/s_i = 1/f - 1/s_o= 1/10 cm - 1/30 cm = (3 - 1)/30 cm.
s_i= 15 cm.
m = -s_i/s_o = -15 cm/30 cm = -1/2.
The image is real, inverted, and diminished in size.
1/s_i = 1/f - 1/s_o= 1/10 cm - 1/15 cm = (3 - 2)/30cm.
s_i = 30 cm.
m = -s_i/s_o = -30 cm/15 cm = -2.
The image is real, inverted, and enlarged in size.
1/s_i = 1/f - 1/s_o= 1/10 cm - 1/5 cm = (1 - 2)/10 cm.
s_i= -10 cm.
m = -s_i/s_o = +10 cm/5 cm = +2.
The image is virtual, erect, and enlarged in size.

14.

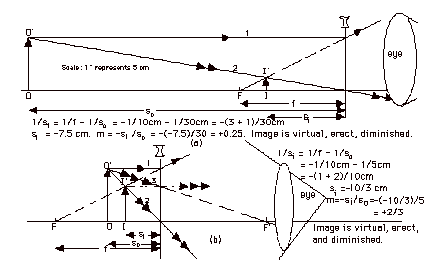
1/s_i = 1/f -1/s_o = 1/10 cm -1/30 cm = - (3 + 1)/30 cm.
s_I = -7.5 cm.
m = -s_i/s_o = - (-7.5 cm)/20 cm = +0.25.
Image is virtual, erect, diminished.
1/s_i = 1/f -1/s_o = 1/10 cm -1/5 cm = - (1 + 2)/10 cm.
s_I = -10/3 cm.
m = -s_i/s_o = - (-10/3 cm)/5 cm = +2/3.
Image is virtual, erect, diminished. See ray diagram in figure above.

15.

With a single converging lens, a virtual image is never inverted, so the image in this problem must be real.

The magnitude of the magnification = 3.0 cm/1.0 cm = s_i/s_o   or
s_i/100 cm = 3 and s_i= +300 cm since it is a real image.   1/s_o + 1/s_i =
1/100 cm + 1/300 cm = 4/300 cm = 1/f.   f = 75 cm.

16.	6.0 cm/2.0 cm = s_i/s_o= s_i/8.0 cm or the magnitude of s_i= 24 cm. Since it is a virtual image, we take s_i= -24 cm. 1/s_o + 1/s_i = 1/8.0 cm - 1/24 cm = (3 - 1)/24 cm = 1/12 cm = 1/f. f = 12 cm.

17.

Magnitude of the magnification = 95/5 =19 = s_i/s_o or 19s_o = s_i (Equation 1)

For the projector, the image is real. s_o + s_i = 4.00 m = 400 cm. (Equation 2)

Substituting Eq. 1 into Eq. 2:
s_o+ 19 s_o= 20 s_o = 400 cm.
s_o= 20 cm; s_i= 380 cm.
1/s_o + 1/s_i = 1/20 cm + 1/380 cm = 20/380 cm = 1/f.
f = 19 cm.

18.	For virtual image, s_i< 0. 1/s_o + 1/s_i = 1/12 cm - 1/6 cm = -1/12 cm = 1/f. f = -12 cm. f is negative, which corresponds to a diverging lens.

19.

Calculate the position of the first image.
1/s_i1 = 1/f₁ - 1/s_o1= 1/2.0cm - 1/2.2cm. s_i1= 22 cm.

For minimum eyestrain, the eyepiece is adjusted so that parallel rays emerge, and hence this image is at the focal point of the eyepiece (Fig. for #19). The total separation of the lenses is 22 cm + 2 cm = 24 cm.
If the object's size is y, the real image formed at F₂ is y’ = y(s_i1/s_o1)
= y(22 cm/2.2 cm) = 10y. Since the eyepiece is adjusted for minimum eyestrain, its magnifying power is given by 25/f = 25/2 = 12.5. The over-all magnifying power of the instrument is the product of the two magnifications = M = (10)(12.5) = 125.

20.	First find the two focal lengths in meters. f_o = 40 cm = 0.40 m 1/f_e= 50 D = 50 m^-1. f_e = 1/50m = 0.02 m. M = f_o/f_e = 0.40/0.02 = 20. This is a low-power telescope, which is desirable when a large area of the sky is to be scanned.

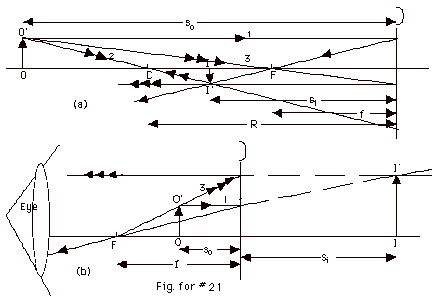
21.

1/s_i = 1/f - 1/s_o = 1/10cm - 1/30cm = (3 - 1)/30cm.
s_i = 15 cm
m = - s_i/s_o = -15/30 = -1/2.
Image is real, inverted, and diminished.
1/s_i = 1/f - 1/s_o = 1/10cm - 1/5cm = (1 - 2)/10cm.
s_i = -10 cm.
m = - s_i/s_o= - (-10cm)/5cm= + 2.
Image is virtual, erect, and enlarged.

In the ray diagrams for the concave mirror, (1) a ray drawn parallel to the principal axis is reflected back through the focal point, (2) a ray drawn through the center of curvature hits the mirror normally (angle of incidence = 0) and is reflected back on itself (angle of reflection = 0), and (3) a ray drawn through the focal point is reflected back parallel to the principal axis.

22.

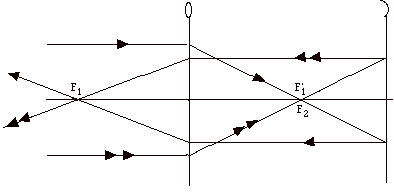
In the figure above, parallel rays incident on the converging lens pass through its focal point F’₁ and the focal point F₂of the concave mirror, hit the mirror, and are reflected back parallel to the principal axis. After returning through the converging lens they pass through its focal point at F₁.

23.

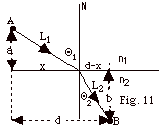
From the geometry of Fig. 11 above,

L₁ + L₂= (a² + x²)^1/2 + [b²+ (d - x)²]^1/2 t = L₁/v₁+ L₂/v₂ t = (a² + x²)^1/2/(c/n₁) + [b²+ (d - x)²]^1/2/(c/n₂)
dt/dx = n₁x/c(a² + x²)^1/2 - n₂(d - x)/c[b²+ (d -x)²]^1/2 For a minimum, dt/dx = 0 or
n₁x/(a² + x²)^1/2 = n₂(d - x)/[b²+ (d -x)²]^1/2
n₁sin Q₁ = n₂ sin Q₂

24.

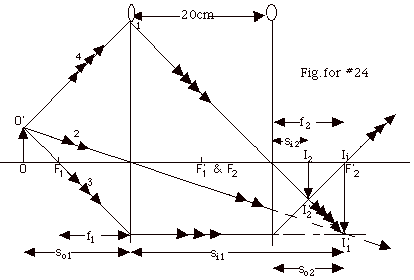
For the first image, 1/s_i1= 1/f₁ - 1/s_o1 = 1/10cm - 1/15cm = (3 - 2)/30cm
= 1/30cm. s_i1= 30 cm. With a distance of 30 cm from the first lens and a lens separation of 20 cm, the image of the first lens (had the second lens not been there) would be formed 10 cm to the right of the second lens. We say this object distance for the second lens is virtual and designate this by taking s_o2 = -10cm. Then 1/s_i2 = 1/f₂ - 1/s_o2 = 1/10cm - (-1/10cm) = 2/10 cm, or s_i2 = 5 cm to the right of the second lens. For the first lens, m₁ = - s_i1/s_o1 = - 30/15 = - 2. For the second lens, m₂ = - s_i2/s_o2 = - 5/(-10) = +1/2. Total magnification = m₁m₂=(-2)(1/2) = - 1.

In Fig. for #24 above, ray 2 is drawn from the top of the object at O’ through the center of the lens. Ray 3 is drawn through the focal point of the first lens F₁ and is refracted through the first lens parallel to the principal axis. If the second lens had not been there, these two rays would meet at the top of the first image I’₁. Any other ray that starts from O’ will also be refracted through the first lens to arrive at I’₁. Ray 4 in Fig. for #24 that passes through the center of the second lens will continue there even with the second lens. (You draw it backwards. Starting at I’₁ draw a line that passes through the center of the second lens until it hits the axis of the first lens. Then draw a line from O’ to the point where it hits the axis of the first lens.) Ray 3 that arrives at the second lens parallel to the principal axis will be refracted through the focal point of the second lens. Rays three and four meet at the top of the second image at I’₂. Thus the second image appears as shown in Fig. for #24 as I₂I’₂. The final image is real (note s_i2 > 0), inverted (first lens inverted the image, but second lens did not invert that image), so the final image is the inverted and the same size as the original object (m₁m₂) = - 1.

25.

1/f_red = (n – 1)(1/R₁ + 1/R₂) = (1.51 – 1)(1/38 cm + 1/38 cm) = 0.0268 cm^-1
1/f – 1/s_o = 0.0268 cm^-1– 1/95 cm = 1/s_i = 0.0163 cm^-1. s_i = 61.3 cm.

1/f_blue = (n – 1)(1/R₁ + 1/R₂) = (1.54 – 1)(1/38 cm + 1/38 cm) = 0.0284 cm^-1
1/f – 1/s_o = 0.0284 cm^-1– 1/95 cm = 1/s_i = 0.0179 cm^-1. s_i = 55.9 cm.

You can buy a camera with an acromatic lens that corrects for the variation in the index of refraction with color. This problem shows the cost may be worth it.

26.

Since we want the farthest point for the eye to be at infinity, we take s_oequal to ∞. Because we need these rays to appear to come from 4.0 m, the far point, we take s_i to be - 4.0 m. The image distance is negative because the rays do not really meet there, but appear to the eye to come from there.

1/s_o + 1/s_i= 1/∞ + -1/4.0m = 1/f. f = - 4.0m. Diopters = 1/f = - 0.25 D.

27.	Now we want the rays to appear to come from 1.25 m when we place an object at the accepted near point of 0.25 m. s_o = 0.25 m and the virtual image position s_i= - 1.25m. 1/s_o + 1/s_i = 1/0.25m + -1/1.25m = 1/f. f = +0.312 m. Diopters = +3.2 m^-1 = + 3.2 D.

Homepage

Sitemap


Website Designed By: Questions, Comments To: Date Created: Date Last Updated:		Susan D. Kunk Phyllis J. Fleming August 8, 2002 March 13, 2003