|
Outline - Simple Harmonic Motion
|
|
- Definition
- Simple harmonic motion occurs when the force F acting on
an object is directly proportional to the displacement x of
the object, but in the opposite direction.
- Mathematical statement F = -kx
- The force is called a restoring force because it always
acts on the object to return it to its equilibrium position.
- Descriptive terms
- The amplitude A is the maximum displacement from the equilibrium
position.
- The period T is the time for one complete oscillation. After
time T the motion repeats itself. In general x(t) = x (t +
T)
- The frequency f is the number of oscillations per second.
The frequency equals the reciprocal of the period. f = 1/T.
- Although simple harmonic motion is not motion in a circle,
it is convenient to use angular frequency by defining w =
2 πf = 2 π/T.
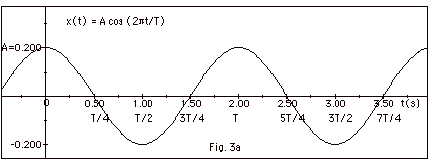
- Example of Simple Harmonic Motion - mass at end of spring
A spring that obeys Hooke's Law is an example of simple harmonic
motion. If you displace the spring a maximum amount x = A, the
amplitude, release it from rest (vo = 0), photograph
and plot the position as function of time, you find, as shown
in Fig. 3a below that x(t) = A cos (2 πt/T),
where T, the period is the time for one complete oscillation.
To save time I shall write:
x(t) = A cos (2 πt/T), as
x(t) = A cos ωt
then,
v(t) = dx/dt = - ωA sin ωt,
as shown in Fig. 3b below:
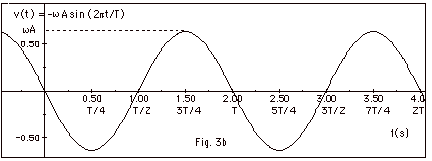
Note: Maximum value of v = ωA
because maximum value of sine =1.
a(t) = dv/dt = - ω2
(A cos ωt), as shown in Fig.
3c below:
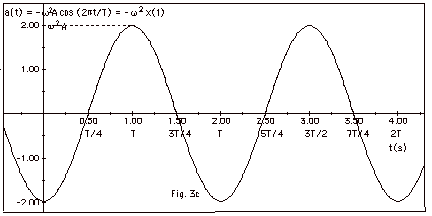
Note: Maximum value of a = ω2A
because maximum value of cosine =1.
a(t) = -[ω2] x(t)
(Equation
2)
|
In general,
|
Fnet = ma
|
|
For a mass attached
to a spring,
|
-kx = ma
|
|
Substituting a
from Equation
2
|
-kx = m[-ω2]
x
|
|
Thus,
|
(k/m) = ω2
|
| |
ω
= [k/m]1/2
|
|
|
f = (1/2 π)[k/m]1/2
|
|
|
T = (2 π)
[m/k]1/2
|
Use Fig. 3 above to:
A. Write x(t) for this graph. First find A, T, f, and ω.
From Fig. 3a, you see that the maximum value of x or the amplitude
A is 0.20 m. The graph repeats itself after one period = T = 2
s, f = 1/T = 0.5 s-1 and ω = 2 πf = π s-1. In general, x(t) = A cos ωt.
For this case x(t) = 0.20 m cos p s-1 t
B. Find a general expression for the velocity, apply it to this
case, and check with Fig. 3b to see if it is correct. What is
the maximum value of the velocity for Fig. 3b? Find x when v =
-0.1 πm/s. Since x(t)
= 0.20 m cos πs-1
t, dx/dt = v(t) = -(0.20 π
m/s) sin π s-1
t. In Fig. 3b we see that v as a function of t is a negative sine
curve with a maximum value of 0.2(3.14) m/s. v = -0.1 π m/s
= -(0.20 π m/s) sin πs-1t. Or 1/2 = sin πs-1t. The sine of an angle is 1/2 when the angle
is 300 or π/6 radians.
So π/6 = π s-1t or t = 1/6 s. x(1/3 s)
= 0.20 m cos π/6= 0.173 cm.
From Fig. 3a and 3b, you can see that these are the correct values.
C. Find a general expression for the acceleration, apply it to
this case, and check with Fig. 3c to see if it is correct. What
is the maximum value of the acceleration for Fig. 3b? Since v(t)
= -(0.20 π m/s) sin π s-1 t, dv/dt = a(t) =
-(0.20 π2 m/s) cos
πs-1 t. In Fig.
3c we see that v as a function of t is a negative cosine curve
with a maximum value of 0.2(3.14)2 m/s2
approximately
equal to 2 m/s2.
D. Sample Problems in 104
Problem Set for Simple Harmonic Motion: 1-6,
10, and 12-16.
- Energy Considerations for Simple Harmonic Motion
- For all cases of simple harmonic motion, that is, when the
force is directly proportional to the displacement of the
object and in the opposite direction F = -kx,
where k is the proportionality constant, the force is a conservative
force and the potential energy function for this force is
U = 1/2 kx2.
- The total energy E equals the sum of the potential energy
U and the kinetic energy K: E = U + K
or
E = 1/2 kx 2 + 1/2 mv2
- When the object has a maximum displacement x = ±A,
its velocity v is zero. E = 1/2 kA2 + 0 Since
E is a constant, E always equals 1/2 kA2 for all
times and displacements.
- When x = 0, U = 0 and E = 0 + 1/2 mv2. The maximum
velocity of the object occurs at the equilibrium position.
- On the next page in Fig. 4, I have drawn the potential energy
curve for the simple harmonic motion shown in Fig. 3. Find
the spring constant k, the mass m of the object and check
out the period of the motion.
- As you can see from Fig. 4, E is approximately equal to
0.20 J. In reality it is equal to 1/2( π2
N/m)(0.20 m)2 = 0.02 π2
J = 1/2 kA2. Since A =
0.20 m, k = π2
N/m. The maximum value of the velocity occurs at the equilibrium
position. We found before that Vmax = ωA
= π s-1 (0.2
m) = 0.2 π m/s. vmax2
= 0.04 π2 m2/s2.
At the equilibrium position, the kinetic energy equals
the total energy =1/2( π2
N/m)(0.20 m)2 = 1/2 mvmax2 =
1/2 m 0.04 π2 m2/s2
(1 N-s2/m) = 1 kg = m.
- Sample Problems in 104
Problem Set for Simple Harmonic Motion: 19,
20.
|
|