Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 104

Outline - Work and Energy
  1. Definition of Work

    1. Work = Fs cos F,s, where F is the force, s is the distance moved and F,s is the angle between F and s. Work is a scalar quantity. Since work is a scalar quantity, you are free to use a + sign for work when the energy of a system increases and a – sign for work when the energy of the system decreases.

    2. Calculation of work done by various forces



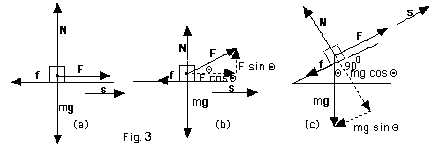
      1. For Fig. 3a above:

        1. Work done by F = Fs cos f,s = Fs cos 0o = Fs
        2. Work done by N = Ns cos N,s = Ns cos 90o = 0
        3. Work done by mg = (mg)s cos mg,s = (mg)s cos -90o = 0
        4. Work done by f = fs cos f,s = fs cos 180o = -fs

      2. For Fig. 3b above:

        1. Work done by F = Fs cos f,s = Fs cos Θ
        2. Work done by N = Ns cos N,s = Ns cos 90o = 0
        3. Work done by mg = (mg)s cos mg,s = (mg)s cos -90o = 0
        4. Work done by f = fs cos f,s = fs cos 180o = -fs

      3. For Fig. 3c above:

        1. Work done by F = Fs cos f,s = Fs cos 0o = Fs
        2. Work done by N = Ns cos N,s = Ns cos 90o = 0
        3. Work done by mg = (mg)s cos mg,s
                                      = (mg)s cos -(Θ + 90o) = -mgs sin Θ
        4. Work done by f = fs cos f,s = fs cos 180o = -fs

    3. Practice problems in 104 Problem Set for Work and Energy: 2-6, 8, 9.


  2. Mechanical Energy

    1. Kinetic Energy K is energy of motion. For an object of mass m moving with a velocity v, K = 1/2 mv2

    2. Potential Energy U is energy of position. Potential energy at point P equals the negative of the work done by a conservative force in going from a point of zero potential to point P.

      1. Near the earth's surface, where the weight of the object is mg, the gravitational potential energy function U = mgh, where m is the mass of the object, g is the acceleration due to gravity and h is the vertical height above the zero potential energy point.

      2. For two point masses, m1 and m2 separated by a distance r, the potential energy of the system is U = -Gm1m2/r. The zero point of the gravitational potential energy for two point particles is infinity.

      3. You will find later that a mass at the end of a spring of constant k, the elastic potential energy function U = 1/2 kx2, where x is the displacement from the equilibrium position.  The equilibrium position, x = 0,  is taken as the zero of potential energy.


    3. Work-energy theorem. The work done by the net force equals the change in the kinetic energy

      1. Example. Each graph in Fig. 4 below describes the one-dimensional motion of a 5.00 kg system during a 4.00 s interval. For each case, what is the work done on the system by the net force during the interval?



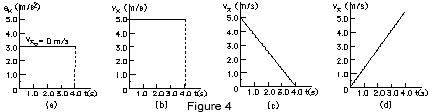
        1. In Fig. 4a, the area under the a versus t graph, the change in velocity = 3.0 m/s2 (4.0 s) = 12 m/s. Work done by net force = change in kinetic energy = 1/2 mvf2 - 1/2 mvo2
          = 1/2(5.0 kg)(12 m/s)2 - 1/2(5.0 kg)(0)2 = 360 J

        2. In Fig. 4b, the velocity is constant. There is no change in kinetic energy and no work is done.

        3. Work done by net force = change in kinetic energy
          = 1/2 mvf2 - 1/2 mvo2
           = 1/2(5.0 kg)(0)2 -1/2(5.0 kg)(5 m/s)2
           = - 62.5 J

        4. Work done by net force = change in kinetic energy
          = 1/2 mvf2 - 1/2 mvo2
          = 1/2(5.0 kg)(5 m/s)2 -1/2(5.0 kg)(0)2
           = +62.5 J

    4. The total energy of a system E = U + K

      1. If no non-conservative force acts on a system, Ef = Ei
        or (Uf + Kf) = (Ui + Ki)

      2. If a non-conservative force acts on the system, work done by the non-conservative force = (Uf + Kf) - (Ui + Ki)

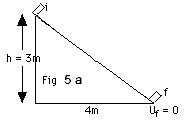
      3. Example: An object of mass m = 2.0 kg is released from rest at the top of a frictionless incline of height 3 m and length 5 m. Taking
        g = 10 m/s2,

        1. use energy considerations to find the velocity of the object at the bottom of the incline

        2. Repeat when µk between the object and the plane is 1/4

      4. Solution:

        1. Since the incline is frictionless and no other nonconservative force acts on the object, energy is conserved. Take the initial point i at the top of the incline and the final point f at the bottom of the incline. Let Uf = 0. At the initial point the potential energy is mgh, where h is the vertical height above the bottom of the incline. The object being released from the top of the incline means that its initial velocity vi is zero so that Ki = 1/2 mvi2 = 0. From conservation of energy, Ui + Ki= Uf + Kf
          2.0 kg(10 m/s2)(3 m) + 0= 0 + 1/2(2.0 kg) vf2
          60 m2/s2= vf2 or vf = (60)1/2 m/s = 7.7 m/s



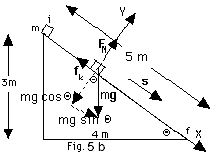
        2. At the bottom of the incline Uf = 0; at top of incline Ui = mgh.
          Ki = 0. With the frictional force, a nonconservative force acting on the block, mechanical energy is not conserved.
          (Fnet)y= may= m(0)
          FN - mg cos Θ = 0
          FN = mg cos Θ
          fk = µkFN = µk mg cos Θ = µk mg(4/5)

          Since the distance down the plane s = 5 m and cos fk,s = 180o, the work by friction = (fk) s cos fk,s
          =          {µk mg cos Θ}s cos 180o
          = (1/4)(2 kg)(10 m/s2)(4/5)}(5m)(-1) = -20 J.

          Work by friction =   (Uf + Kf)    -    (Ui + Ki)
                      20 J = (0 + 1/2 mvf’2) - (mgh + 0)
                     -20 J = 1/2(2 kg)vf’2    - (2 kg)(10 m/s2)(3 m)
                     -20 J = 1 kg vf’2           - 60 J
          40 J = 40 N - m = 40 kg m2/s2 = vf’2 kg
          vf’ = (40)1/2 m/s = 6.3 m/s



    5. Practice problems in 104 Problem Set for Work and Energy: 10-23.


  3. Scalar or Dot Product




    1. Definition C = A • B = |A||B|cos symbol for right angleA, B

    2. Work = Fs cos symbol for right angleF,s may be written as F • s

    3. Unit vectors

      1. i • i = |i||i|cos symbol for right anglei, i = |1||1|cos 0o = 1

        j • j = |j||j|cos symbol for right anglej, j = |1||1|cos 0o = 1

        k • k = |k||k|cos symbol for right anglek, k = |1||1|cos 0o = 1

      2. i • j = |i||j|cos symbol for right anglei, j = |1||1|cos 90o = 0 = j • i

        i • k = |i||k|cos symbol for right anglei, k = |1||1|cos 90o = 0 = k • i

        j • k = |j||k|cos symbol for right anglej, k = |1||1|cos 90o = 0 = k • j


  4. Practice problem in 104 Problem Set for Work and Energy: 17.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
September 25, 2002
April 3, 2003