|
Review - Center of Mass, Momentum,
and Collisions
|
|
|
1.
|
A tennis player receives a shot with
the ball (0.060 kg) traveling horizontally at 50 m/s, and
returns the shot with the ball traveling horizontally at 40
m/s in the opposite direction. What is the impulse delivered
to the ball by the racket?
|
|
|
2.
|
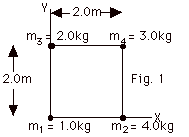
Four particles are distributed in the X-Y plane as shown in Fig. 1 below. Find the coordinates (XCM, YCM) of the particles.
The particles are on the corners of a 2.0-m square.
|
|
|
|
3.
|
A 1500-kg automobile travels eastward at a speed of 8.0
m/s. It makes a 900 turn to the north in a time
of 3.0 s and continues with the same speed. Find (a) the impulse
delivered to the car as a result of the turn (b) the average
force exerted on the car during the turn and (c) the average
force of the car on the road during the turn.
|
|
|
4.
|
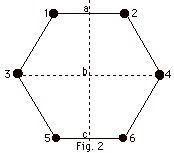
Six particles with equal mass m are arranged
to correspond to the six corners of a regular hexagon, as
shown in Fig. 2 below. Locate the center of mass of the system.
|
|
|
|
5.
|
Object A of mass 1.0 kg travels with a speed of 4.0 m/s, while object B of mass 2.0 kg travels with a
speed of 2.00 m/s. Compare (a) the magnitude of their momenta
and (b) their kinetic energies.
|
|
|
6.
|
Two
skaters stand in the center of a circular rink of frictionless
ice. When the skaters push on one another, they move apart
along the same straight line. If the skater of mass m1
= 50 kg reaches the edge of the rink after 12 s and
the other skater reaches the opposite edge of the rink in
18 s, what is the mass m2 of the second skater?
|
|
|
7.
|
A 0.050-kg block moving to the right
with a speed of 0.20 m/s collides with a 0.10-kg block moving
to the left with a speed of 0.04 m/s (Fig.3 below). After
the collision the blocks stick together and go off with a
common velocity vf.
Find vf.
|
|

|
|
8.
|
Find the position of the center of mass of five equal-mass
particles located at the five corners of a square based right
pyramid with sides of length L and height h.
|
|
|
9.
|
A 75-kg man is standing in a 225-kg barge
and is originally 10 m from a pier on a still lake. The man
walks towards the pier a distance of 2.0 m relative to the
pier, and then stops. Assuming the barge can move through
the water without resistance how far is the man now from the
pier?
|
|
|
10.
|
After a nucleus decays, the products of the decay move
off in opposite directions with equal and opposite momenta.
What is the initial momentum of the nucleus?
|
|
|
11.
|
Object A with mass mA = 3.0
kg moving in the +x-direction with a velocity of
1.0 m/s collides with object B with mB = 2.0 kg
moving in the +y-direction with velocity of 2.0 m/s. After
the collision, the blocks stick together. Find the magnitude
and direction of the final velocity vf.
|
|
|
12.
|
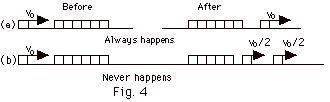
Seven steel blocks of equal mass m rest
on a frictionless table. If one of the blocks is given an
initial velocity vo and it collides
with the other six, a block at the other end always goes off
with vo (Fig. 4a below). The collision
in Fig. 4b never happens. Suggest a reason why the collision
in Fig. 4b never happens.
|
|
|
|
13.
|
On a frictionless table, a 3-kg block
moving 5 m/s to the right collides with a
2-kg block moving 10 m/s to the left. If the blocks collide
and stick together after the collision, find their final velocity.
|
|
|
14.
|
This is a one-dimensional problem. A
1.0-kg block moving with a velocity of
2.0 m/s to the right collides elastically with a second block
that is initially at rest. Find the velocities of the two
blocks after the collision if the mass of the second block
is (a) 1.0 kg, (b) 1,000 kg, (c) 0.001 kg.
|
|
|
15.
|
A disk with a mass m1 = 10 kg traveling along
a smooth surface with a velocity of 24 m/s along the +X-axis
collides with a stationary second disk of mass m2 =
20 kg. After the collision, the first disk moves along the
+ Y-axis with a velocity of 10 m/s. What is the velocity (direction
and magnitude) of the second disk after the collision?
|
|
|
16.
|
A disk with a mass m1 = 2.0 kg traveling
along a smooth surface with a velocity of 39 m/s along the
+X-axis collides with a stationary second disk of mass m2
= 8.0 kg. After the collision the second disk moves
with a velocity of 10 m/s at an angle of 370 above
the X axis. (a) Find the velocity (magnitude and direction)
of the first disk after the collision. (b) Is this an elastic
collision?
|
|
|
17.
|
A proton moving with a velocity of v1i i collides elastically with another proton initially at
rest. If both protons have the same speed (v1f
= v2f) after the collision, find (a) the speed of each proton after the collision in terms
of v1i and (b) the direction of the velocity vectors
after the collision.
|
|
|
18.
|
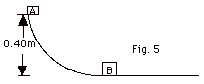
A 2.0-kg Block A slides down a frictionless
chute (Fig. 5 below) and then strikes a stationary 9.2-kg
block B on a horizontal table. (a) What is the velocity of
A at the bottom of the chute? (b) If A sticks to B after the
collision, what is their common velocity immediately after
the collision? (c) If the frictional force between the blocks
and the table is 2.8 N, how far do they slide before coming
to rest? Take g = 9.8 m/s2.
|
|
 |
|
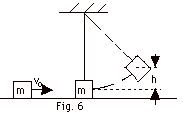
19.
|
A mass m hangs by a string of length
L. A second identical mass is sliding along a frictionless
surface with velocity vo (Fig. 6
below). (a) If the two masses have an elastic collision, how
high will the first mass rise? (b) If the two masses have
a totally inelastic collision, how high will the masses rise?
|
|
 |
|
20.
|
A horizontal force of 0.80 N is required
to move m2 = 5.0 kg of Fig. 7 below
across the surface with a constant velocity. With the block
initially at rest, a 0.020-kg bullet m1
fired horizontally into the block causes the block (with
bullet inside) to slide 1.5 m before coming to rest again.
Find the speed vo of the bullet.
|
|
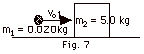 |
|
21.
|
A 2.0-kg steel ball A and a 3.0-kg steel
ball B coated with putty are suspended by strings of length
L = 0.80 m. When ball A is released from the position shown
in Fig. 8 below, it strikes ball B. After the collision, the
balls move with a common velocity. Find (a) the kinetic energy
of ball A just before the collision, (b) the velocity of ball
A just before the collision, (c) the common velocity of the
balls immediately after the collision, and (d) the height
to which the two balls rise after the collision. Take g =
10 m/s2.
|
|
 |
|
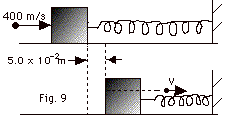
22.
|
A 5.0 x 10-3-kg
bullet moving with an initial speed of 400 m/s is fired into
and passes through a 1.0-kg block, as shown in Fig. 9 below.
The block initially at rest on a frictionless, horizontal
surface is connected to a spring of force constant 900 N/m.
If the block moves a distance of 5.0 x 10-2
m to the right after impact and comes to rest momentarily,
find (a) the speed at which the bullet emerges from the block
and (b) the energy lost in the block.
|
|
 |
|
23.
|
A number of years ago, The New Yorker
gave the following quote,“Every time you drop a pin,
the earth moves upward slightly.” The comment after
that was, "Butterfingers." Please explain the quote.
|
|