|
Answers - Center of Mass, Momentum,
Collisions
|
|
|
1.
|
Impulse J = pf - pi
= mvf - mvi. Taking the return direction
as positive,
J = 0.060 kg{(40 - (-50)}m/s = 5.4 kg-m/s.
|
|
|
2.
|
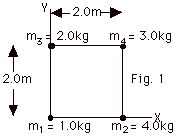
XCM = (m1x1 + m2x2
+ m3x3 + m4x4)/(
m1 + m2 + m3 + m4)
= {(1.0 kg)(0) +
(4.0 kg)(2.0 m) + (2.0 kg)(0) + (3.0 kg)(2.0 m)}/(10.0 kg)
= 1.4 m
YCM = (m1y1 + m2y2
+ m3y3 + m4y4)/(
m1 + m2 + m3 + m4)
= {(1.0 kg)(0) +
(4.0 kg)(0) + (2.0 kg)(2.0 m) + (3.0 kg)(2.0 m)}/(10.0 kg)
= 1.0 m
|
|
|
3.
|
Taking east in the +i direction
and north in the +j direction,
(a) J = pf - pi =
(1500 kg)(8.0 m/s)(j - i) = 12 x 103
kg-m/s(j - i)
(b) J = Fav t Fav = J/t
= {12 x 103 kg-m/s(j - i)}/3.0 s
=4 x 103 N(j - i)
(c) By Newton's third law of motion, the average force of
the car on the road
= -4 x 103 N(j -
i)
|
|
|
4.
|
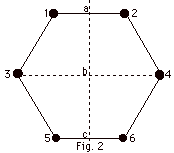
Divide the system into three pairs of particles, consisting
of 1 + 2, 3 + 4, and
5 + 6. The midway for 1 + 2 is at a and the midpoint for 5
+ 6 is c. We can treat 1 + 2 as a point particle of mass of
2m at a and 5 + 6 as a point particle of mass 2m at c. We
can combine these two point particles to be another point
particle with mass 4m at b. 3 + 4 have their midpoint at b
so we can replace them with a point particle of mass 2m at
b. Finally the whole works acts like a point particle of mass
6m at b.
|
|
|
5.
|
(a) pA = (1.0 kg)(4.0 m/s)
= 4.0 kg-m/s
pB = (2.0 kg)(2.0
m/s) = 4.0 kg-m/s
(b) KA = 1/2(1.0 kg)(4.0 m/s)2 = 8.0
J
KB = 1/2(2.0 kg)(2.0
m/s)2 = 4.0 J
While the momenta are equal, the kinetic energies are not.
|
|
|
6.
|
Take the distance to the edge of the
rink as x. Then the velocity v1 after the collision
for the skater of mass m1 equals +x/12 s and the
velocity v2 of the other skater equals -x/18 s.
From conservation of momentum, pi = pf,
0 = (50 kg)(x/12 s) + (m2)(-x/18 s), or (m2)(x/18
s) = (50 kg)(x/12 s).
m2 = (50 kg)((18/12) = 75 kg.
|
|
|
7.
|
Take to the right to be positive. From
conservation of momentum,
|
pi
|
=
|
pf
|
|
0.050 kg(0.20 m/s) + (0.10 kg)((-0.04
m/s)
|
=
|
(0.05 + 0.10)kg vf
|
|
(0.010 - 0.004)kg-m/s
|
=
|
(0.15 kg)vf
|
| |
|
|
|
vf
|
=
|
0.040 m/s to the right
|
| |
|
|
|
|
|
8.
|
The center of mass of the four particles
at the base of the pyramid is the center of the base. The
center of mass of the five-particle system lies on the line
perpendicular to the base and passes through the peak of the
pyramid. This line passes through the center of mass of the
four particles at the base and gives the x-coordinate of the
center of mass at the center of the base.
YCM = {(m)(h) + (4m)(0)}/5m = h/5.
|
|
|
9.
|
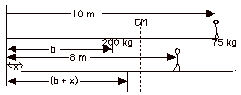
In the figure above, b represents the center of mass of the
barge and x the distance the barge moves to the right as the
man moves to the left. No external forces act on the barge-man
system and the center of mass CM of this system remains at
rest.
Initially,
XCM = {(75 kg)(10 m) +
(200 kg)(b)}/(75 + 200)kg (Equation
1)
Finally,
XCM = {(75 kg)(8 m) + (200
kg)(b + x)}/(75 + 200)kg (Equation
2)
Since the CM of the system remains at rest, equate Eq. 1 and
Eq. 2 and cancel denominators:
|
(75 kg)(10 m) + (200 kg)(b) = (75
kg)(8 m) + (200kg)(b + x) (3)
|
|
75 kg(10 - 8)m = 200 kg x
|
|
150 m = 200 x
|
|
x = 0.75 m
|
|
|
|
10.
|
Momentum is conserved:
Since the final momentum of product 1, p1f, =
-p2f, the negative of the final momentum
of product 2, the initial momentum pin of
the nucleus must equal zero.
|
|
|
11.
|
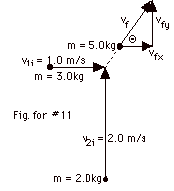
|
(pi)x
|
=
|
(pf)x
|
|
|
(3.0 kg)(1.0 m/s)
|
=
|
(5.0 kg)(vf)x
|
(vf)x
= 0.60 m/s
|
| |
|
|
|
|
(pi)y
|
=
|
(pf)y
|
|
|
(2.0 kg)(2.0 m/s)
|
=
|
(5.0 kg)(vf)y
|
(vf)y
= 0.80 m/s
|
| |
|
|
|
|
vf = {(0.60)2 +
(0.80)2}1/2 = 1.0 m/s. tan
Θ = 0.8/0.6
|
|
Θ =530.
|
|
|
|
|
|
|
12.
|

|
pi
= pf:
|
mvo
|
=
|
mvo
|
|
Ki
= Kf
|
1/2 mvo2
|
=
|
1/2 mvo2
|

|
pi
= pf
|
mvo
|
=
|
mvo/2 + mvo/2
|
|
Ki ≠ Kf
|
1/2 mvo2
|
≠
|
1/2 m(vo/2)2 +
1/2 m(vo/2)2
|
Another conservation law is at work here.
In an elastic collision, kinetic energy is also conserved.
For an elastic collision, (b) cannot occur.
|
|
|
13.
|
Conservation of momentum:
| |
pi
|
=
|
pf
|
| |
(3 kg)(5 m/s) + (2 kg)(-10m/s)
|
=
|
(3 + 2)kg vcommon
|
| |
15 kg-m/s - 20 kg-m/s
|
=
|
5 kg vcommon
|
| |
vcommon
|
=
|
-1 m/s
|
After the collision, the blocks move
to the left with a speed of 1 m/s.
|
|
|
14.
|
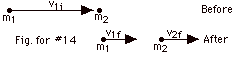
For an elastic collision,
| |
pi
|
=
|
pf
|
| |
m1v1i
|
=
|
m1v1f + m2v2f
(Equation
1)
|
where I have assumed both final velocities
to the right. In any calculation, if one of them is to the
left, you will get a minus answer in your solution.
| |
Ki
|
=
|
Kf
|
| |
1/2 m1v1i2
|
=
|
1/2 m1v1f2
+ 1/2 m2v2f2 (Equation
2)
|
Solving Eq. 1 and Eq. 2 simultaneously
for v1f and v2f gives:
v1f = {(m1 - m2)/(m1
+ m2)} v1i, and
v2f = {2m1/(m1 + m2)}
v1i
For v1i = 2.0 m/s, m1 = 1.0 kg, and
(a) m2 = 1.0 kg, v1f = 0 and v2f
= 2.0 m/s = v1f.
Example: cue ball colliding with another billiard ball.
(b) m2 = 1000 kg, v1f = -(999/1001)(2.0
m/s) ≈(1)(2.0 m/s) = -v1i.
v2f = (2.0/1001)(2.0 m/s) ≈ 0.004 m/s, which
is small compared to v1i.
A basketball colliding with a bowling ball is an example of
a relatively small mass colliding with a large mass.
(c) m2 = 0.001 kg, v1f = (0.999)(2.0
m/s) ≈ (1)(2.0 m/s) = v1i.
v2f = (2.0/1.001)(1.0 m/s) ≈ 2 v1i.
A bowling ball colliding with a basketball is an example of
a relatively large mass colliding with a small mass.
|
|
|
15.
|
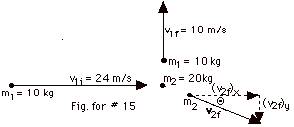
If momentum, a vector quantity is conserved, its components
are conserved.
| |
(pi)x = (pf)x
|
| |
10 kg(24 m/s) = (20 kg)(v2f)x
|
| |
( v2f)x =
12 m/s
|
| |
(pi)y = (pf)y
|
| |
0 = 10 kg(10 m/s)+20 kg (v2f)y
(v2f)y = -5.0 m/s
|
| |
v2f = {(v2f)x2
+ (v2f)y2}1/2
|
| |
v2f = (122
+ -5.02)1/2 m/s = 13 m/s
|
| |
tan Θ
= -5/12
|
| |
Θ =
-22.60
|
| |
|
| |
|
|
|
|
16.
|
| |
(pi)x = (pf)x
cos 370 = 0.80 and sin 370
= 0.60
|
| |
2.0 kg(39 m/s) = 2.0 kg(v1f)x
+ 8.0 kg(10 m/s)(0.80)
|
| |
(78 - 64)m/s = 2.0(v1f)x.
(v1f)x = 7.0 m/s
|
| |
|
| |
(pi)y =
(pf)y
|
| |
0 = 2.0 kg(v1f)y
+ 8.0 kg(10 m/s)(0.6)
|
| |
-24 m/s = (v1f)y
|
| |
|
| |
v1f = {(7.0)2
+ (-24)2}1/2 m/s = 25 m/s
|
| |
tan Θ
= -24/7
|
| |
Θ =
-820
|
|
|
|
17.
|
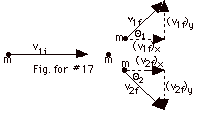
Momentum is conserved.
| |
|
(px)i = (px)f
|
| |
|
mv1i = m(v1f)x
+ m(v2f)x (Equation
1)
|
| |
|
(py)i = (py)f
|
| |
|
0 = mv1f sin >Θ1
+ mv2f sin Θ2
|
| |
Since
|
v1f = v2f
|
| |
|
Θ1
= Θ2
|
| |
and
|
(v1f)x =
(v2f)x (Equation
2)
|
| |
|
|
So from Eq. 1, v1i =
2 (v1f)x = 2v1f cos Θ1. (Equation
3)
From conservation of kinetic energy,
|
Ki
|
=
|
Kf
|
|
1/2 m v1i2
|
=
|
1/2 m v1f2
+ 1/2 m
|
|
v2f2
|
=
|
mv1f2
|
|
or
v1f = v2f = v1i/√2
|
From Eq. (3),
v1i = 2 (v1i/√2) cos Θ1
cos Θ1 = √2/2,
and
Θ1 = 450
Θ2 = -450
|
|
|
18.
|
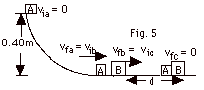
(a) Take the zero of gravitational potential energy at the
bottom of the ramp, Uaf = 0. At the top of the
ramp, Uai = mgh, where h = 0.40 m. On the ramp,
from conservation of energy,
|
Uaf
|
+
|
Kaf
|
=
|
Uai
|
+
|
Kai
|
|
mA(9.8 m/s)(0.40 m)
|
+
|
0
|
=
|
0
|
+
|
1/2 mAvfa2
|
|
vfa = [19.6(0.4)]1/2m/s
= 2.8 m/s
|
(b) When A collides with B, momentum
is conserved.
|
pbi
|
=
|
pbf
|
|
mAvib
|
=
|
(mA + mB)vfb
|
|
vfb = [mA/(mA
+ mB)]vib = [2.0/(11.2)]2.8 m/s
= 0.50 m/s
|
(c) Work done by friction = (Ucf
+ Kcf ) - (Uci + Kci)
fd
cos 1800 = (0 + 0) - [0 - 1/2(11.2 kg)(0.50 m/s)2]
-(2.8
N)d = -1.4 N-m and
d = 0.50 m.
|
|
|
19.
|
|
(a) For an elastic collision in
one dimension,
|
v1f = (m1
- m2)/(m1 + m2)
v1i, and
|
|
v2f = 2m1/(m1
+ m2) v1i
|
In this problem, m1
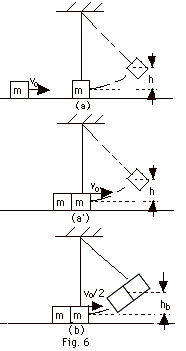
= m2 and v1i = vo. Thus
the first block stops after the collision, v1f
= 0, and the second block gains a velocity v2f
= vo. (Fig. 6a to the right) Now the second
block swings up to height h. Energy is conserved. Taking
the middle of the block as the zero of potential energy
at the initial position i, the potential energy at "final"
position of height h, where the block comes momentarily
to rest is mgh.
|
Ui
|
+
|
Ki
|
=
|
Uf
|
+
|
Kf
|
|
0
|
+
|
1/2 mvo2
|
=
|
mgh
|
+
|
0
|
|
and
h = vo2/2g
|
(b) For the inelastic
collision,
| |
|
pi
|
=
|
pf
|
|
|
| |
|
mvo
|
=
|
2mvb
|
|
|
| |
|
vb
|
=
|
vo/2
|
|
|
| |
|
|
|
|
|
|
|
Uib
|
+
|
Kib
|
=
|
Ufb
|
+ |
Kfb
|
|
0
|
+
|
1/2(2m)(vo2/4)
|
=
|
2mgh
|
+ |
0
|
|
and
hb = vo2/8g
|
|
 |
|
|
|
20.
|
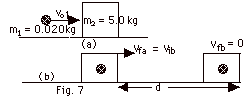
| |
pia
|
=
|
pfa
|
| |
0.020 kg(vo1)
|
=
|
(0. 020 + 5.0)kg vfa
|
| |
vfa
|
=
|
(0.02/5.02)vo1
|
It takes 0.80 N to keep the 5.0 kg moving
with constant velocity. The increase in the normal force due
to the weight of the bullet does not change the frictional
force to two significant figures. Take the gravitational potential
at the surface = 0 = Ufb = Uib.
| |
Work by friction
|
=
|
(Ufb + Kfb)
|
-
|
(Uib + Kib)
|
| |
-(0.80 N)(1.5 m)
|
=
|
(0 + 0)
|
-
|
{0 + 1/2(0.502 kg)[0.02vo1/5.02]2}
|
| |
-1.2 kg(m/s)2
|
=
|
-3.98 x 10-6 vo12
kg
|
| |
vo1
|
=
|
549 m/s
|
|
|
|
|
|
21.
|
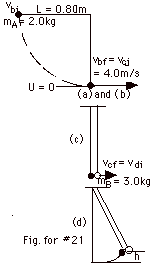
Take the potential energy at the bottom of swing to be zero.
The kinetic energy at the top of the swing is zero since the
velocity of A there vbi = 0. See the figure for
#21, above, for parts (a), (b), (c) and (d).
(a) Ubf + Kbf = Ubi + Kbi
0 +
Kbf = (2.0kg)(10 m/s2)(0.80 m) + 0
Kbf
= 16 J = 1/2(2.0 kg)vbf2.
(b) vbf = 4.0 m/s
(c) pi = pf 2.0 kg(4.0 m/s) = (2.0 +
3.0)kg vcf. vcf = 1.6m/s.
(d) Udf
+ Kdf = Udi + Kdi
5.0 kg(10 m/s2)h
+ 0 = 0 + 1/2(5.0 kg)(1.6m/s)2
h
= 0.13 m
|
|
|
22.
|
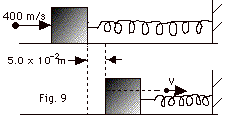
(a) Do the problem in steps. First there is the collision
between the bullet of mass m = 5.0 x 10-3 kg travelling
with a speed of 400 m/s and the block of mass M = 1.0 kg.
After the collision, the bullet has a velocity v and the block
a velocity vM. From conservation of momentum,
|
pi
= pf
|
|
(5.0 x 10-3 kg)(400
m/s) = (1.0 kg)vM + (5.0 x 10-3
kg)v (Equation
1)
|
Just after the collision and just before
the block compresses the spring, the initial potential energy
of the block-spring system is 0 and the kinetic energy of
the block is 1/2 (1.0 kg) vM2. When
the spring has been compressed to its maximum, the block momentarily
comes to rest and its "final" kinetic energy is
zero, but the potential energy of the block-spring system
is 1/2 kx2 =
1/2(900 N/m)((5 x 10-2m)2. From conservation
of energy,
|
Ui
+ Ki = Uf + Kf
|
|
0 + 1/2(1.0 kg) vM2
= 1/2(900 N/m)(5.0 x 10-2 m)2
+ 0
|
|
or
vM = 1.5 m/s
|
Substituting this value of vM
back into Eq. 1:
(5.0 x 10-3 kg)(400m/s) = (1.0kg)(1.5m/s)
+ (5.0 x10-3 kg)v or v = 100 m/s.
(b) For the collision,
| |
Ki = 1/2 (5 x 10-3
kg)(400 m/s)2 = 400 J |
|
|
Kf = 1/2(5.0 x 10-3
kg)(100 m/s)2 + 1/2(1.0 kg)(1.5 m/s)2
= (25 + 1.12)J |
|
|
K lost = (400 - 26.12)J = 374 J |
|
|
|
23.
|
This problem is a reminder to choose
your system carefully. When you drop an object, its momentum
does not stay constant because the gravitational attraction
of the earth, an external force, acts on it. If you take your
system to be the object and the earth, then momentum is conserved.
Let the object be a textbook with mass mb = 2.0
kg and velocity vb. The earth has a mass Me
= 5.98 x 1024 kg. Before you release the book,
the momentum of the system is zero.
pi = pf
0 = mbvb + Meve,
where ve = the speed acquired
by the earth
ve = (mb/Me)vb
= (2.0 kg/5.98 x 1024 kg)vb = 3.3 x
10-25 vb
So the earth does move up, but we do not detect the motion.
|
|