Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 107

Answers - Work and Energy

1.


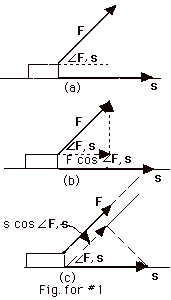
Work equals the force F times the displacement s times the cosine of the angle F,s between the force F and the displacement s:
W = (Fs cos F,s)
If we rewrite work as
W = (F cos F,s)s
we see from Fig. 1b above that we may say, alternatively, that work is the component of the force, F cos F,s, in the direction of the displacement times the displacement. From Fig. 1c above we find we may also write
W = F (s cos F,s)
or work is the product of the force and the component of the displacement,
(s cos F,s), in the direction of the force.

2.



In general, W = (Fs cos F,s)
  1. For the girl, W =18 N(4 m)(cos 0o) =18 N(4 m)(1) = 72 J

  2. For the boy, W = 12 N(4 m)(cos 180o) = 12 N(4 m)(-1) = -48 J

  3. You can find the work done by the net force in two ways:

    1. Since work is a scalar quantity, add the work done by each algebraically, that is, 72 J - 48 J = 24 J.

    2. First find the net force. Taking to the right to be positive,

      Fnet = 18 N - 12 N = 6 N to the right
      Fnet, s = 0o
      Work done by net force = 6 N(4 m) cos 0o = 6 N(4 m)(1) = 24 J

3.


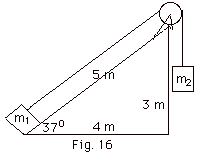
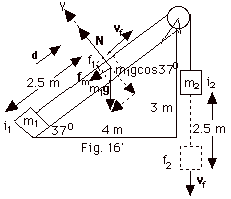
If you do not raise the object or increase its velocity, there is no increase in the object’s potential energy or in its kinetic energy. The physicists’ definition of work demands that when you do work on an object its energy increases. For the situation in Fig. 1 above, you are exerting a force through a distance, but you do no work. For this reason, we define work W as the force F times the displacement s times the angle between F and s or F,s. In Fig. 1, F,s = 90o and cos 90o = 0. With this definition of work, we correctly predict that no work is done by moving an object horizontally without increasing its speed. You may tire while moving the object because your tensed muscles are continually contracting and relaxing in minute movements. You, however, have done no work on the object as defined in a physical sense.

4.

The force that produces a centripetal acceleration is always in toward the center or perpendicular to the path. The angle between the tension and the displacement is 90o. Since cos 90o = 0, no work is done by the tension in the string. Also note that the potential energy and the kinetic energy of the object does not change.

5.


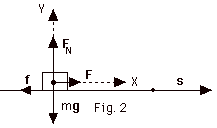
  1. The angle between F and s is 0o.
    The work done by F = Fs cos 0o = 12 N(4 m)(1) = 48 J.

  2. The angle between FN and s is 90o.
    The work done by FN = FNs cos 90o = FNs(0) = 0.

  3. The angle between mg and s is -90o.
    The work done by mg = mgs cos -90o = mgs(0) = 0.

  4. To find the frictional force f, notice that:

    (Fnet)y = may = m(0) = 0
    FN - mg = 0 and FN = mg
    f = µkFN = 0.50(mg) = 0.50(20 N) = 10 N.

    The angle between f and s is 180o.
    The work done by f = fs cos 180o = 10 N(4 m)(-1) = - 40 J.

  5. The work done by the net force = 48 J - 40 J = 8 J  or
    (Fnet)x = 12 N - 10 N = 2 N.
    Work done by net force = 2 N(4 m)cos 00 = 8 J.

6.

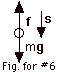For the raindrop to fall with a constant speed, the net force acting on the drop must equal 0. Thus mg - f = 0 or mg = f, where f is the frictional force of the air on the drop and I have taken down as positive. Work = Force(displacement) F,s. The gravitational force mg is in the same direction as the displacement s.

Work by gravitational force equals:
mgs cos 0o = mgs = 3.35 x 10-5 kg(10 m/s2)(100 m)(1) = 3.35 x 10-2 J.
The frictional force of the air is opposite to the direction of the displacement s. Work by frictional force of air = fs cos 180o = -3.35 x 10-2 J.

7.

 Kinetic energy K = 1/2 mv2 = 1/2(52 kg)(14.0 m/s)2 = 51 x 102 J.

8.
  1. v • v = (v)(v) cos v,  v = v2 cos 0o = v2(1) = v2.

  2. d(v • v) = v • dv + dv • v = 2v • dv because the order of the vectors in a dot or scalar product does not matter. The scalar product is commutative. d(v2) = 2v dv.  Since v • v = v2, d(v • v) = d(v2) = 2v dv.

9.
  1. Work done by net force =
    (Fnet) x cos 00 = (ma)x.     (Equation 1)

    For motion along the x axis with constant acceleration,
    vf2 = vo2 + 2ax  or
    ax = 1/2(vf2 - vo2)         (Equation 2).
    Substituting ax from Eq. 2 into Eq. 1: Work done by net force
    = m{1/2(vf2 - vo2)}
    = 1/2 mvf2 - 1/2 mvo2
    = change in kinetic energy.


  2. ∫ Fnet • ds = ∫ m dv/dt • ds = ∫ m dv/dt • v dt = m∫ v • dv

    In the above equation, we have used the definition ds = v dt and the result of #8 that v • dv = v dv.

10.

 Work done by net force = 8 J = 1/2 (2.0 kg)vf2 - 1/2 (2.0 kg)vo2.
8 J = 8 N-m = 8 kg(m/s2)(m) = 1 kg vf2 - 1/2 (2.0 kg)(0)  or  vf = 2(2)1/2 m/s.

11.

F, ds = Θ and ds cos Θ = dy, as shown in Fig. 3 above.  F . ds = (-mg)dy.
The work done in going from A to B .


12.

 From work energy theorem,
Work done = 1/2 mvB2 - 1/2 mvA2
From #11,
mgh = 1/2 mvB2 - 1/2 mvA2
vB = (2gh + vA2)1/2

13.


  1. In the figure, we take the Y-axis perpendicular to the incline.

    (Fnet)y = may
    FN - mg cos 37o = m(0)
    FN = mg cos 37o = (2.0 kg)(10 m/s2)(0.8) =16 N
    f = µkFN = 0.50(16 N) = 8.0 N

  2. Work done by F = Fs cos F,s = 30N(4m)(cos 0o) = 30 N(4.0 m)(1) =120 J.

  3. Work done by FN = FNs cos 90o = FNs(0) = 0.

  4. Angle between mg and s = -(90 + 37)o.
    Work done by mg = mgs cos mg,s
    =     2.0 kg(10 m/s2)(4.0 m)cos {-127o)}
    = 2.0 kg(10 m/s2)(4.0 m) (-0.6) = -48 J.

  5. Work done by friction = f(s)cos 180o = 8.0 N(4.0 m)(-1) = -32 J

  6. Work by net force =120 J - 32 J + 0 - 48 J = 40 J.

  7. By work-energy theorem, work done by net force =
    40 J = ΔK = 1/2 mvf2 - 1/2 mvi2 = 1/2(2kg)vf2 - 0.
    40 J = 40 N-m = 40 (kg-m/s2)-m = 40 kg-m2/s2 = (kg)vf2.
    vf = (40)1/2 m/s.

14.

  1. For the Y-axis, (Fnet)y = may.  From the figure,

    FN - F sin 37o - mg = 0
    FN = F sin 37o + mg
    FN = (30 N)(0.6) + 20 N = 38 N.
    f = µkFN = (0.50)(38 N) = 19 N

  2. Work by f = fs cos f, s = (19 N)(4.0 m)(cos 180o) = -76 N

  3. Work done by F = Fs cos F,s = 30 N(4.0 m)(cos 37o) =120 J(0.8) = 96 J

  4. Work done by FN = FNs cos FN,s = FNs cos 90o = FNs (0) = 0

  5. Work done by mg = mgs cos mg, s = mgs cos (-90o) = mgs (0) = 0.

  6. Net work done = Work by f + Work by F + Work by FN + Work by mg                         = -76 J + 96 J + 0 + 0 = 20 J

  7. By work energy theorem,
    Work done by net force = Δkinetic energy = Kf - Ki = 1/2 mvf2 - 1/2 mvi2
                                   20 J = 1/2 (2 kg)vf2 - 0
    vf = (20 J/kg)1/2 = (20 N-m/kg)1/2 = {20 (kg-m/s2)(m/kg)}1/2
        = √20 m/s = 2√5 m/s.

15.

 Work = force(displacement) cosF,s
  1. The angle between the force exerted by the person and the displacement is 0o.  Work by person =10 N(1.0 m) = 10 J.

  2. The gravitational force = mg = 1.0 kg(10 m/s2) = 10 N. To lift the object with a constant velocity the force of the person must be equal in magnitude to the gravitational force. The angle between mg and s is 180o. Work done by gravity = 10 N(1.0 m)cos 180o = -10 J.

  3. Increase in potential energy = mgh, where h is the height to which the object is raised = 10 J. The increase in the potential energy equals the work done by the person in raising the object with a constant velocity or it equals the negative of the work done by the gravitational force.

  4. The increase in kinetic energy is zero because the work done by the net force is zero.

16.
  1. Now work done by person = 12 N(1.0 m) = 12 J.

  2. Work done by gravitational force still equals -10 J.

  3. Increase in potential energy equals the negative of the work done by the gravitational force = 10 J.

  4. Work done by the net force = 12 J - 10 J = 2 J = increase in kinetic energy.

17.
  1. Work done by friction = fs cos 180o= 72 N(3.0 m)(-1) = -216 J.

  2. Work by net force = work done by friction = change in kinetic energy

    -216 J = 1/2 (12 kg)v23.0m - 1/2(12 kg)(10 m/s)2
    -216 J + 600 J = 1/2(12 kg)v23.0m.
    V3.0m = 8.0 m/s2.

  3. Work done by friction = 72 N(s)(-1) = change in kinetic energy = 0 - 600 J.
    s = 8.3 m

18.


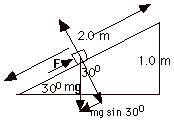
For the figure above,
  1. sine of the angle of incline = 1.0/2.0.  Angle of incline = 30o.  For motion of the object with constant velocity, the net force in that direction must be zero.

    Fnet up the plane = ma = m(0)
    F - mg sin 30o = 0    Force of person =
    F = mg sin 30o = 2.0 kg(10 m/s2)(1/2) = 10 N.

    The angle between the force of the person and the displacement is 0o. Work done by person in moving the object up the plane of 2.0 m with a constant velocity = 10 N(2.0 m)(cos 0o) = 10 N(2.0 m)(1) = 20 J.

  2. The component of the force of gravity down the plane is
    mg sin 30o = 10 N.
    The angle between this component and the displacement up the incline is 180o.  The work done by the gravitational force =
    (10 N)(2.0 m)(-1) = -20 J.
    The work done by the net force = work done by person + work done by gravitational force = increase in kinetic energy. Work done by person =
    increase in kinetic energy - work done by gravitational force
    = {1/2 (2.0 kg)(3.0 m/s2)2 - 0} - (-20 J) = 29 J.

  3. Again, Fnet up the plane = ma = m(0) = 0, but now there is a frictional force of 3.0 N so that F - mg sin 30o - 3.0 N = 0, or F = 13 N.  Work done by person = 13 N(2.0 m)(1) = 26 J.

19.


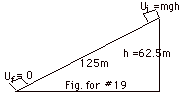
Take Uf at the bottom of the incline = 0.
Work done by friction = fs cos 180o = (Uf + Kf) - (Ui + Ki).
f(125 m)(-1) = {0 +(1/2)(1.0 kg)(25 m/s)2} - {1.0 kg)(10 m/s2)(62.5 m) + 0}.
-125 m f = 312.5 J - 625 J = -312.5 J.
f = 2.5 N


20.

 The total energy E = U + K  or  K = E - U = {-21.7 - (-43.4)}10-19 J.
K = 21.7 x 10-19 J.  While the total and potential energy are negative, classically the kinetic energy must always be positive.

21.
  1. Acceleration a = Δv/ Δt = (0.20 - 0)m/s/0.50 s = 0.40 m/s2.
    F = ma = 2000 kg(0.40 m/s2) = 800 N.

  2. K = 1/2(2000 kg)(0.20 m/s)2 = 40 J.

  3. Work done by net force = change in kinetic energy = 40 J.

  4. Work = Fs = 800 N s = 40 J.   s = 40 J/800 N = 0.05 m.

22.
  1. i . i = (1)(1) cos 0o = 1

  2. i . j = j . i = (1)(1) cos 90o = 0

  3. j . j = (1)(1) cos 0o = 1

  4. W = F . s = 20 N j . 4 m i = 80 J(j . i) = 0

  5. W = F . s= (3i + 4j)N . (2i - 2j)m
    = 6J(i . i) - 6J(i . j) + 8J(j . i) - 8J(j . j)
    = 6 J - 0 + 0 - 8 J = -2 J

23.


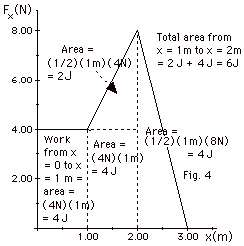
Work done = ∫Fx dx = area under curve.  I have added the calculations of the areas to Fig. 4 above.  As shown in the figure,
  1. the work done from x = 0  to x = 1.0 m is 4.0 J,

  2. the work done in going from x = 1.0 m to x = 2.0 m is 2.0 J + 4.0 J = 6.0 J, and

  3. the work done in going from x = 2.0 m to x = 3.0 m is 4.0 J.  From the work-energy theorem the work done equals the change in kinetic energy.

  4. From x = 0  to x = 1.0 m, work done = 4.0 J = 1/2 mv12 - 0, since vo = 0. 4.0 J = 1/2(2.0 kg)v12  or  v1 = 2.0 m/s.

  5. From x = 1.0 m  to x = 2.0m, work done = 6.0 J = 1/2 mv22 - 1/2 mv12
    = 1/2(2.0 kg)v22 - 1/2(2.0 kg)(4.0 m2/s2)  or  6.0 J = 1 kg v22 - 4.0 J.
    6.0 J + 4.0 J = 10 J = 10 kg(m/s)2 = 1.0 kg v22
     and  v2 = (10)1/2 m/s.

  6. From x = 2.0 m to 3.0 m, work done = 4.0 J = 1/2 mv32 - 1/2 mv22
    4.0 J = 1/2(2.0 kg)v32 - 10 J
    14 J = 1/2(2.0 kg)v32  and  v3 = (14)1/2 m/s.

24.


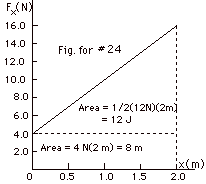
dW = F . ds = (4.0 N + 6.0 N/m x)i . dx i = (4.0 N + 6.0 N/m x)dx since i . i = 1.


You can also find the work done from the area under the curve of Fx as a function of x, as is shown in the Fig. for #24 above.

25.


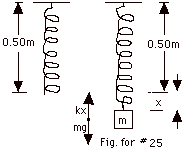
When the mass m = 0.50 kg is attached to the spring and it comes to rest,
Fnet = ma = m(0).

For up positive, kx - mg = 0 or (50 N/m)x - (0.50 kg)(10 m/s2) = 0
and x = 5.0 N/(50 N/m) = 0.10 m.

The new equilibrium length = (0.50 + 0.10)m = 0.60 m.

26.
  1. Fnet = ma
    T = mv2/r = (1.0 kg)(1.0 m/s)2/1.0 m = 1.0 N

  2. The tension equals the force due to the spring = kx = 1.0 N,
    where x = R - relaxed length = 1.0 m - 0.9 = 0.1 m.
    k = 1.0 N/x = 1.0 N/0.1 m = 10 N/m

  3. Now kx’ = mv’2/R’  or  (10 N/m)(R’ - 0.9 m) = 1.0 kg(2.0 m/s)2/R’ or
    10 N/m (R’)(R’ - 0.9 m) = 4.0 N-m
    10 R’2 - 9 R’ m - 4.0 m2 = 0.
    R’ = {9m ± (81 m2 + 160 m2)1/2}/20 = 1.23 m.

  4. Work to increase the kinetic energy of the mass
    = 1/2 m(v’2 - v2)
    = 1/2 (1.0 kg)(4.0 - 1.0)m2/s2 = 1.5 J

    x’ = R’ - R = 1.23 m - 1.00 m = 0.23 m

    Work to stretch spring
    = 1/2 k(x’2 - x2)
    = 1/2(10 N/m)(0.053 - 0.010) = 0.21 J.

    Total work done = (1.5 + 0.2)J = 1.7 J

27.
  1. Fc . ds = -kx2 i . dx i = -kx2 dx.


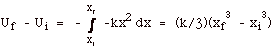
  2. Let xi = 0  and  Ui = 0,  xf = x  and  Uf = U.
    Then U(x) = 1/3 kx3.

28.


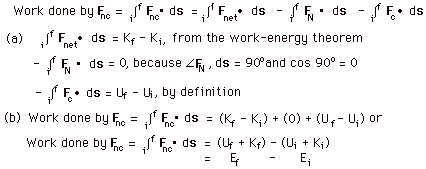
      where E = the total mechanical energy.

 (c) If the nonconservative force Fnc = 0,

Ef = Ei          and
Uf + Kf = Ui + Ki


29.


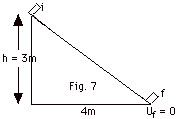
Since the incline is frictionless and no other nonconservative force acts on the object, energy is conserved. Take the initial point i at the top of the incline and the final point f at the bottom of the incline. Let Uf = 0. At the initial point the potential energy is mgh, where h is the vertical height above the bottom of the incline. The object being released from the top of the incline means that its initial velocity vi is zero so that  Ki = 1/2 mvi2 = 0.  From conservation of energy,
                             Ui + Ki = Uf + Kf
2.0 kg(10 m/s2)(3 m) + 0 = 0 + 1/2(2.0 kg) vf2
                          60 m2/s2 = vf2  or
                                    vf = (60)1/2 m/s = 7.7 m/s

30.


At the bottom of the incline Uf = 0 and Ui = mgh.  Ki still = 0.  With the frictional force, a nonconservative force acting on the block, mechanical energy is not conserved.

(Fnet)y = may = m(0)
FN = mg cos Θ
fk = µkFN = µk mg cos Θ = µk mg(4/5)

Since the distance down the plane s = 5 m and cos fk,s = 180o, the work by friction =
(fk) s cos fk, s=
{µk mg cos Θ}s cos 180o
= (1/4)(2 kg)(10 m/s2)(4/5)}(5m)(-1)=-20 J.
Work done by friction =
                       (Uf + Kf) - (Ui + Ki)
-20 J = (0 + 1/2 mvf’2) - (mgh + 0)
-20 J = 1/2(2 kg)vf’2 - (2 kg)(10 m/s2)(3 m) = 1kg vf’2 - 60 J
40 J = 40 N-m = 40 kg m2/s2 = vf’2 kg
vf’ = (40)1/2 m/s = 6.3 m/s

31.

 Now take at bottom of incline, Ui = 0 and Ki = 60 J.

After the spring has maximum compression,
Uf = 1/2 kx2 =1/2(480 N/m)x2  and
Kf = 0.

0 + 60 J = 240 N/m x2 + 0.     x2 = 1/4 m2  and  x = 1/2 m.

32.


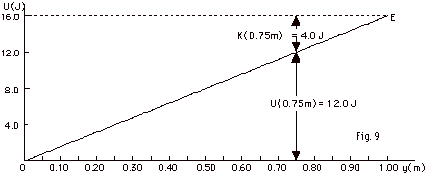
  1. From Fig. 9 above, at y = 0.750 m,  U = 12.0 J.

  2. Since at all times E = 16.0 J = U + K = U(0.750m) + K(0.750 m) =
    12.0 J + K(0.750 m)  or  K(0.750m) = 4.0 J.

  3. U = mgh  or,  at 0.750 m, 12.0 J = m(10 m/s2)(0.75 m)  or  m = 1.6 kg.

  4. K = 1/2 mv2 = 1/2(1.6kg)v2 = 4.0 J  or  v = (5)1/2 m/s.

33.


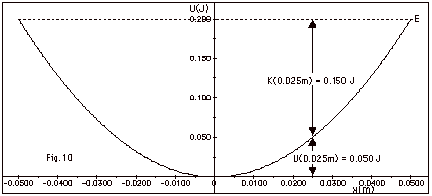
  1. From Fig. 10 above, at x = 0.025 m,  U = 0.050 J.

  2. E = 0.200 J = U + K = 0.050 J + K.   K = 0.150 J.

  3. U = 0.050 J = 1/2 kx2= 1/2 k(0.025 m)2  or
    k = 0.10 J/6.25 x 10-4 m2 = 160 N/m.

  4. K = 0.150 J = 1/2 mv2 = 1/2(0.30kg)v2  or
    v2 = 2(0.150)/0.30 m2/s2 = 1.0 m2/s2  or  v = 1.0 m/s.

  5. E = 0.200 J =1/2 kxmax2  = 1/2 (160 N/m)xmax2   or
    xmax = (25 x 10-4)1/2m = 0.05m.

  6. v is a maximum when the potential energy is a minimum.
    This occurs for x = 0  and, therefore,  U = 0
    with K = E = 0.200 J = 1/2 mvmax2 = 1/2(0.30kg)vmax2.
    vmax = 1.15 m/s.

34.


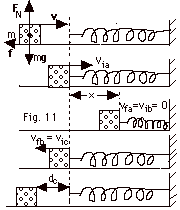
  1. (Fnet)y = may = m(0) = 0
    FN - mg = 0 or FN = mg
    f = µkFN = µkmg

    Work done by f = fs cos f, s = fx cos 180o
     = µkmgx(-1) = -0.30(1.0 kg)(10 m/s2)x

    Work done by friction = (Uaf + Kaf) - (Uai + Kai)
    -3.0x N = (1/2 kx2 + 0) - (0 + 1/2 mvia2)
    -3.0xN = 1/2 (10N/m x2) - 1/2 (1.0 kg)(16 m2/s2)
    -3.0x m = 5.0x2 - 8.0 m2.    5.0x2 + 3.0x m - 8.0 m2 = 0
    x = {-3.0 m ± (9 m2 +160 m2)1/2}/10 and x = 1.0 m

  2. Work done by friction = (Ubf + Kbf) - (Ubi + Kbi)
    -3.0xN = (0 + 1/2 mvbf2) - (1/2 kx2 + 0)
    -3.0(1.0 m)N = 1/2(1.0 kg)vbf2 - 1/2(10N/m)(1.0 m)2
     -3.0 J = 1/2 kg vbf2 - 5.0 J  or  2.0 J = 1/2 kg vbf2  and
    4.0(m/s)2 = vbf2
    vbf = 2.0 m/s

  3. Work done by friction = (Ucf + Kcf) - (Uci + Kci)
    -3.0dcN = (0 + 0) - (0 + 1/2 mvci) = -1/2 (1.0 kg)(4.0 m2/s2) = -2.0 J
    dc = 2/3 m

35.

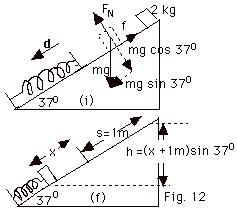
  1. I took the gravitational potential energy = Ugf = 0 at the final position.

  2. This makes the initial gravitational potential energy (see Fig. 12f above) equal mgh, where h = (x + 1 m)sin 37o.
    Ui = 20N(x + 1m)3/5 = 12N(x + 1m).

  3. Ki = 0 because the block is released from rest.

  4. In Fig. 12i above, taking the Y-axis perpendicular to the plane,

    (Fnet)y = may
    FN - mg cos 37o = m(0) = 0  or
    FN = mg cos 37o = 20 N (4/5) = 16 N
    f = µFN = 1/8(16 N) = 2.0 N.

  5. While in the final position, the gravitational potential energy is zero, the spring potential energy = 1/2 kx2 =1/2(120 N/m)x2 = 60 N/m x2.

  6. When the spring has maximum compression, the block comes to rest
    so Kf = 0.

  7. Work done by frictional force = f . d = fd cos 180o = -(2N)(x + 1 m).

  8. Work done by           f             =      (Uf + Kf) - (Ui + Ki)
    -2.0 N(x + 1m) = (60 N/m x2 + 0) - {(12N)(x + 1m) + 0}
    10 N(x + 1m) = 10x N + 10J = 60 N/m x2  or
    6.0 x2 - 1.0x m -1.0 m2 = 0   or
    x2 - (1/6) x m - 1/6 m2    
    and  (x - 1/2 m)(x + 1/3 m) = 0
    or   x = 1/2 m    

                                                                                             

36.


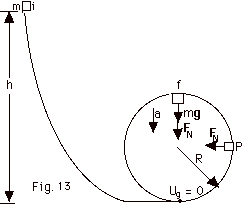
  1. We use conservation of energy to solve the problem. We take the gravitational potential energy equal to 0 at the bottom of the loop-the-loop (Fig. 13 above). The block is released at the initial position i so the kinetic energy there is zero. The block will make it around the loop-the-loop if it can stay on the loop as it gets to the top of the loop. For this reason we take the top of the loop as the final position f.
       Ui + Ki = Uf + Kf
    mgh + 0 = 2mgR + 1/2 mvf2  or
    mgh = 2mgR + 1/2 mvf2                 (Equation 1)
    At the top, the acceleration is in toward the center.
          Fnet = ma       or
    mg + FN = mvf2/R
    For the minimum height h, we want the minimum velocity vf,  so we set the normal force FN = 0.  Then,
    mg = mvf2/R  or  mvf2 = mgR      (Equation 2)

    Substituting Eq. 2 into Eq. 1:
    mgh = 2mgR + mgR = 5/2 mgR  or  h = 5R/2

  2. At P, the potential energy = mgR.
                Ui + Ki = UP + KP  or
    mg(5/2 R) + 0 = mgR + 1/2 mvP2  or
    mg(3/2)R =1/2 mvP2  or
    mvP2 = 3mgR
    At P, the normal force produces the centripetal acceleration into the center of the circle.  At P, mg is down.
    Fnet = ma
    FN = mvP2/R = {3mgR}/R = 3mg

37.


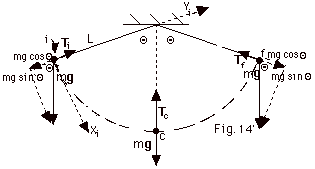
In Fig. 14’ above, I show the forces acting on the bob. One force is the tension in the string. This force is always in toward the center of the arc. The other force is the weight of the bob mg. At point i and f, mg is neither away from the center of the circle nor tangent to the arc, so we take components of mg. At c, T is up and mg is down. In Fig. 14’ at the initial point i, I have drawn Xi and Yi axes. I take the Y axis in the direction of the centripetal acceleration. There is also an acceleration in the X direction, because there is an increase in the magnitude of the velocity. Later we shall refer to this as the tangential acceleration.
At i                   (Fnet)y = may
  Ti - mg cos Θ= mvi2/L = m(0)/L,
because the object is at rest at i. Then, Ti = mg cos Θ. If you look at Fig. 14’, you see that Tf = mg cos Θ because vf also equals zero. In both cases, for the tangential direction,
    (Fnet)x = matangential   or
- mg sin Θ= matangential
At c, Tc is in toward the center of the circle and mg is away from the center. Taking the positive direction as that of the centripetal acceleration in toward the center of the circle,
    Fnet = ma
Tc - mg = mvc2/L   or
        Tc = mg + mvc2/L         (Equation 1)



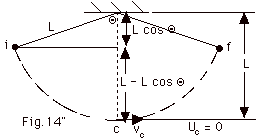
Clearly to find Tc ,  we must find vc.  We turn to conservation of energy. We set the potential energy = 0 at the lowest point so that Uc = 0. The method of finding the height of point i above c is shown in Fig. 14” immediately above. L is the hypotenuse of the triangle in Fig. 14” and the side adjacent is L cos Θ. The height of i above c is then L - L cos Θ = L(1 - cos Θ).  From conservation of energy,
                   Ui + Ki = Uc + Kc
mgL(1 - cos Θ) + 0 = 0 + 1/2 (m)(vc)2  or
                  mvc2/L = 2mg(1 - cos Θ)         (Equation 2)
Substituting Eq. 2 into Eq. 1,
Tc = mg + 2mg(1 - cos Θ) = mg(3 - 2 cos Θ)

38.


  1. Start with conservation of energy because we are told the surface is frictionless.
                 Ui + Ki = Ut + Kt .     Taking Ui = 0,
    0 + 1/2(mvo2) = 2mgR + 1/2 mvt2,

    where t is at the top of the circle, a distance of 2R above i.

                  mvo2 = mvt2 + 4mgR      (Equation 1)
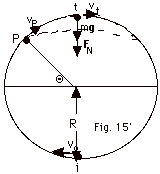
    What does it mean that "m makes it around the circle"? If it makes it by the highest point t, everything is swell. Look at the forces acting on m at the highest point (Fig. 15’ above). At t with the net force and the acceleration into the center of the circle, down is positive.
          Fnet = ma
    mg + FN = mvt2/R

    The minimum value of vt and vo  is for FN = 0  or
          mgR = mvt2    (Equation 2)
    Substituting the value of mvt2  from Eq. 2 into Eq. 1,
    mvo2 = mgR + 4mgR = 5mgR   or
    vo = (5gR)1/2





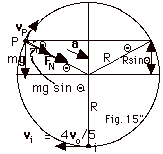
  2. Now we look at energy conditions at P and the forces acting on the object at P. We draw a new diagram, to show the forces at P and decide the conditions that allow the object to lose its circular path. Just before the object departs from its path, the normal force goes to zero. The conditions are shown in Fig. 15” immediately above. The two forces acting on the object at P are the weight mg and the normal force FN. There must be a net force in toward the center of the circle to change the direction of the velocity. The normal force FN is perpendicular to the surface and inward, but mg is neither radially inward nor tangent to the circle. We must find the component of mg in to the center of the circle. From Fig. 15”, we see this is mg sinΘ.  The centripetal acceleration is also in this direction.
    (Fnet) in to center = ma
    mg sin Θ + FN = mvP2/R
    When the object leaves the surface, FN = 0
    and  mgR sin Θ = mvP2      (Equation 3)

    We don't know the value of Θ or vP,  so we turn to conservation of energy.

    From (a) we know that vo = (5gR)1/2.
    We take vi = 4vo/5 = (4/5)(5gR)1/2.
            So vi2 = (4/5)2 {(5gR)1/2}2 = (16/25)(5gR).

    As shown in Fig. 15”,  the vertical height of point P above i is:
                      (R + R sin Θ) = R(1 + sin Θ).
                               Ui + Ki = UP + KP
     0 + 1/2(m)(16/25)(5gR) = mgR(1 + sin Θ) + 1/2 mvP2   (Equation 4)
    Substituting Eq. 3 into Eq. 4 and doing a little arithmetic, we find:
    8mgR/5 = mgR + mgR sin Θ + (1/2)mgR sin Θ  or
    8mgR/5 - 5mgR/5 = 3mgR/5 = 3mgR sin Θ/2
    so sin Θ= 2/5  and  Θ = 24o.

39.

We choose different potential energy positions for the potential energy equal to zero for m1 and m2.  Notice that when m2 moves down,  m1 moves up the incline. Since we always take the lowest position for the zero of potential energy,  the position of  m1  in Fig. 16  (above left)  is its lowest position and we take the potential energy of  m1  equal to zero there. The position of  m2  in Fig. 16 is its highest position. Thus we take its zero potential at a point 2.5 m below where it is in Fig. 16 or its dashed position in Fig. 16’  (above right).  Mechanical energy is not conserved.

(Fnet)y = m1ay
 FN - m1g cos 37o = m1(0) = 0   or
FN = m1g cos 37o = m1g(4/5).
f = µFN = 0.55(m1g)(4/5) =
0.55(2.0 kg)(10 m/s2)(4/5) = 8.8 N.

The work done by friction = f(d) cos f,d = fd cos 180o
= -fd = -8.8 N(2.5 m) = -22 J.

In the final position f2,  the potential energy (U2)f of m2 is zero, but in the final position f1,  the potential energy for m1 is m1gh1,  where h1 = the vertical height above the initial position. Since it goes up the plane 2.5 m and the plane is inclined at 37o,  the vertical height h1 = 2.5 m sin 37o = 2.5 m (3/5) =1.5 m.

(U1)f = (m1g)h1 = (20 N)1.5 m = 30 J.  For the system Uf = (U1)f + (U2)f = 30 J + 0 = 30 J.  Since both blocks move together, their final speed vf is the same. The final kinetic energy of the system Kf = (1/2)(m1 + m2)vf2 = 1/2(6.0 kg) vf2.  The initial potential energy (U1)i  of  m1  at  i1  is zero. The initial potential energy of  m2  at  i2  is (U2)i = m2g(2.5 m) = (4.0 kg)(10 m/s2)(2.5 m) = 100 J.  For the system,  Ui = 100 J.  Both blocks were initially at rest.  For the system Ki = 0. Again,

Work by friction = { Uf  +        Kf       } - {  Ui    + Ki }
       -22 J           = {30 J + 3.0 kg(vf2)} - {100 J + 0 }
-22 J - 30 J + 100 J = 48 J = 48 N-m = 3.0 kg vf2
48 kg-m2/s2 = 3.0 kg vf2   or
vf2 =16 m2/s2  and  vf = 4 m/s

40.
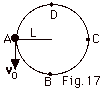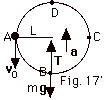  1. Take UB = 0 and use conservation of energy:
      UA           +          KA        =          UD       +       KD
    mgL           +   1/2(m)vo2   =       mg2L      +        0       or
                           1/2(m)vo2   =       mgL    or  vo = (2gL)1/2
    Note: If the ball were attached to a string, it could not go up to D and stop. A string can only pull in toward the center of a circle so at the top of the circle, the gravitational force would cause the ball to fall down. On the other hand, a rod can provide a force up or down, so when it comes to rest, the upward force of the rod balances the gravitational force and the ball returns on its path clockwise.

  2. At B (Fig. 17’, above right) the acceleration is up so take up as positive.
    Fnet = ma
    T - mg = mvB2/L               (Equation 1)
    Turn to conservation of energy to find vB.
    UA       +       KA             =          UB       +          KB
     mgL     + 1/2(m)vo2       =          0         +    (1/2)mvB2
    From (a),
    mgL + (1/2)m{(2gL)1/2}2 = 0 + (1/2)mvB2   or
    mgL +           mgL              = (1/2)mvB2   or
    mvB2 = 4mgL         (Equation 2)
    Substituting Eq. 2 into Eq. 1,
    T - mg = 4mgL/L = 4mg   and   T = 5mg

  3. Work done by frictional force
    = (UC + KC) - (UA + KA)
    = (mgL + 0) - (mgL + mgL)
    = mgL - 2mgL
    = - mgL

  4. Work done by frictional force
    = (UB + KB) - (UA + KA)
    = (0 + 0) - (mgL + mgL)
    = - 2mgL

41.

In our calculations, we are assuming the peg is so small (even if it is drawn a little larger so you can see it) that the mass of the rope is essentially that of a rope of length 0.60 m on the right of the peg and 0.40 m on the left of the peg. The mass per unit length = m/L.  The mass of length dy = (the mass per unit length)dy = (m/L)dy.




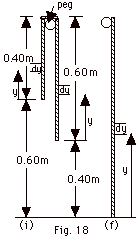
In Fig. 18 above, we take the gravitational potential energy equal to zero at the point where the right end of the rope hits the ground shown by the horizontal line at the bottom of the figure. In Fig. 18i, the initial potential energy of a length dy on the right side of the peg is:

(mass/length dy)(g)(y + 0.40 m) = (m/L)dyg(y + 0.40 m)
One on the left side is:
(mass/length dy)(g)(y + 0.60 m) = (m/L)dyg(y + 0.60m)
For the entire rope,


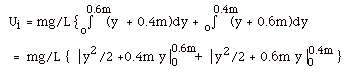
(0.20 kg)(10 m/s2)/(1.0 m){0.36 m2/2 + 0.24 m2 + 0.16 m2/2 + 0.24 m2}
= 1.48 J.

When the rope just touches the ground (Fig. 18f), the potential energy
= Uf = (mg/L){ o∫1.0m y dy = (0.20 kg)(10 m/s2/(1.0 m){1.0 m2/2}
= 1.00 J.

Since there is no friction, the total mechanical energy is conserved.                     
    Ui   + Ki  =   Uf    +    Kf
1.48 J + 0  = 1.00 J + 1/2(0.20 kg)vf2
vf2 = (1.48 -1.00)J/0.10 kg = 4.8 (kg m2/s2)/(kg)
vf = 2.2 m/s

42.


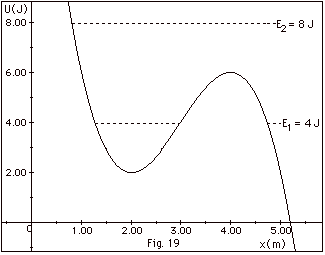
  1. A particle initially at x = 2.0 m and 2.0 J < E < 6.0 J would be trapped and undergo back-and-forth motion. For example for E = 4.0 J,  the particle would go back and forth between x = 1.27 m and 3.0 m. See (e) below.

  2. A particle initially at x = 5.0 m for 2.0 J < E < 6.0 J could move to lower values of x until about x = 4.75 m, then it would experience a repulsive force because F4.75 m =  - (dU/dx)at x = 4.75 m > 0, and go off to higher values of x.

  3. A particle initially at x = 2.0 m and moving to smaller values of x for
    E > 8.0 J  experiences a repulsive force, escapes from the potential well, and moves off forever to the right.

  4. The potential energy at x = 3.00 m is 4.00 J.  E = U + K.  8.0 J = 4.0 J + K.
    K = 4.0 J = 1/2 mv2 = 1/2(2.0 kg)vf2.
    vf = (4.0 J/kg)1/2 = (4.0 kg-m2/s2/kg)1/2 = 2.0 m/s.

  5. At x =1.27m (Fig. 19’ below),



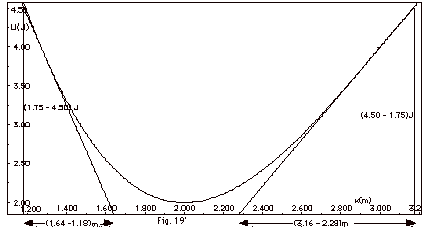
    1. - slope = - (1.75 -4.50)J/(1.64 -1.18)m = 6.00 N.
    2. - dU/dx = -3.00 J/m +3m-1(x m-1 - 3)2 J.
      (-dU/dx)x = 1.27m = -3.00 N + 3m-1(1.27 - 3)2 J = 6.00 N.

  6. At x = 3.00 m,

    1. - slope = - (4.50 - 1.75)J/(3.16 - 2.28)m = -3.12 N.
    2. (-dU/dx)x = 3.00m = - (3.00 N ) + (3)(3 -3)2N = -3.00 N.
      3.12 is within the drawing error of 3.00.

43.


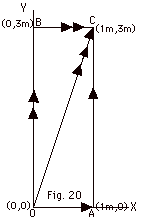
dW = F . ds = 2.0 N/m3(xy2 i +yx2 j)(dx i + dy j)
                   = 2.0 N/m3(xy2 dx + yx2 dy)

 because i . i = j . j = 1  and  i . j = j . i = 0.
  1. From O to A,  y = dy = 0  and  W = 0.
    From A to C,  dx = 0  and  x = 1.0 m,
    W =(2.0 N/m3) o∫3.0m y(1.0 m2)dy = 9.0 J.

  2. From O to B,  x = dx = 0  and  W = 0.
    From B to C,  y = 3.0 m  and  dy = 0.
    W =(2.0 N/m3) o∫1.0m x(9.0 m2)dx = 9.0 J.

  3. From O to C,  y = 3.0x  and  dy = 3.0dx,
    xy2 dx = x(3.0x)2dx = 9.0x3 dx  and
    yx2 dy = (3.0x)x2(3.0dx) = 9.0x3 dx.
    W = 36 N/m3 o∫1.0m x3 dx = 9.0 J.

  4. From O to A to C, W = +9.0 J.
    From C to 0, W = - 9.0 J.
    From O to A to C to O,  W = 0.

  5. The force appears conservative. The work is independent of path and equals zero for a closed path.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
October 8, 2002
April 16, 2003