|
Outline - Simple Harmonic Motion
|
|
- Definition of Simple Harmonic Motion
- Simple harmonic motion occurs when the force F acting
on an object is directly proportional to the displacement
x of the object, but in the opposite direction.
- Mathematical statement F = - kx
- The force is called a restoring force because it always
acts on the object to return it to its equilibrium position.
- Descriptive terms
- The amplitude A is the maximum displacement from the equilibrium
position.
- The period T is the time for one complete oscillation. After
time T the motion repeats itself. In general x(t) = x (t +
T).
- The frequency f is the number of oscillations per second.
The frequency equals the reciprocal of the period. f
= 1/T.
- Although simple harmonic motion is not motion in a circle,
it is convenient to use angular frequency by defining w
= 2pf = 2p/T.
- Example of Simple Harmonic Motion - mass at end of spring
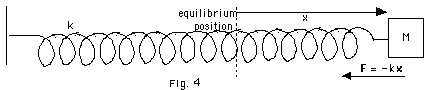
- When the object is at its equilibrium position, the spring
is neither stretched or compressed.
- Differential equation of motion
- Fnet = ma
- kx = ma
- Since v = dx/dt, and
a = dv/dt, a = d(dx/dt)/dt = d2x/dt2
- kx = md2x/dt2 or
d2x/dt2 +(k/m)x
= 0
(Equation
1)
- Solution of Equation 1 gives x as a function of t.
If you have had differential equations, you can solve
this equation. If not, the following equation can be shown
to be true by experiment. In any event the solution to
Equation 1 is:
x(t) = A cos (2pt/T
+ F) = A cos (2pft
+ F) = A (cos wt
+ F)
(Equation
2)
- Verification of A and T in Equation 2
- The maximum value of cos (2pt/T
+ F) = 1. Thus
the maximum value of x = A, which is the definition
of A.
- The value of x(t) must equal the value of x(t +
T) if the motion repeats itself after one period.
Thus for this to be true,
x(t) = x(t + T)
x(t + T) = A cos [(2pt
+T)/T + F]
= A cos [2pt/T
+ 2p + F]
= A cos [2pt/T
+ F]
= x(t)
since 2p= 360o
and cos Q =
cos (Q + 2p)
so x(t) = x(t + T)
- Finding an expression for w.
From Equation 2,
- v(t) = dx/dt = -wA
sin (wt + F)
- a(t) = dv/dt = d2x/dt2 = -w2
[A cos (wt + F)
= -w2
(x)
- Substitute d2x/dt2 = -w2
(x) into Equation 1:
-w2
(x) + (k/m)(x) = 0, or
w = (k/m)1/2
- For mass-spring, x(t) = A cos [(k/m)1/2
t + F]
- Sample problems in 107
Problem Set for Simple Harmonic Motion: 1-4,
11, 12.
- Phase angle F and initial
conditions
- x(t) = A (cos wt +
F). x(0) = xo
= A cos F. cos
F =xo/A
v(t) = -wA sin (wt
+ F). v(0) = vo =
-wA sin F.
sin F = -vo
/wA
- tan F = sin F/cos
F = (-vo
/wA)/(xo/A)
= -vo /wxo
- sin2 F
+ cos2 F =
(-vo /wA)2
+ (xo/A)2
1 = (1/A2)[ (-vo /w)2
+ (xo)2]
A2 = [ (-vo /w)2
+ (xo)2], or
A = [(xo)2 + (-vo /w)2]1/2
- Special Cases
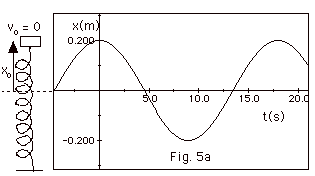
Find x(t) for w = 0.350
Hz = 0.350 s-1
- When xo = 0.20 m and vo = 0
A = [(xo)2 + (-vo /w)2]1/2
= [(0.20 m)2 + 0]1/2 = 0.20 m
cos F =xo/A=0.20
m/0.20 m = 1
sin F = -vo /wA
= 0. F= 0.
x(t) = 0.20 m cos 0.350 s-1t.
Fig. 5a below shows x as a function of t with the mass
at xo = A, vo = 0, and F
= 0.
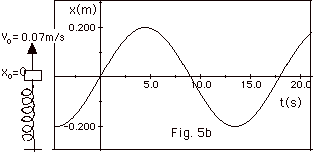
- When xo = 0 and vo = 0.070 m/s
A = [(xo)2 + (-vo /w)2]1/2
= {(0) + [(-0.070m/s)/0.35s-1]2}1/2
= 0.20 m
cosF =xo/A
= 0/0.20 m = 0.
sinF = -vo /wA
= -0.070m/s/(0.350s-1)(0.20m) = -1. F=
-p/2.
x (t) = 0.20 m cos (0.350 s-1t - p/2)
A plot of x as a function of t for xo = 0,
vo = 0.07 m/s
with F = - p/2 is
shown in Fig. 5b below.
- When xo = 0.1(3)1/2 m and vo
= 0.035 m/s
A = [(xo)2 + (-vo /w)2]1/2
=
{(0.03) + [(-0.035m/s)/0.35s-1]2}1/2
= 0.20 m
tan F = -vo /wxo
= -0.070m/s/(0.350s-1)(0.1√3 m) = -0.577
F= -p/6
x (t) = 0.20 m cos (0.350 s-1t - p/6)
A plot of x as a function of t for xo = 0.1(3)1/2
m, vo = 0.035 m/s with F
= - p/6 is shown in Fig. 5 c below.
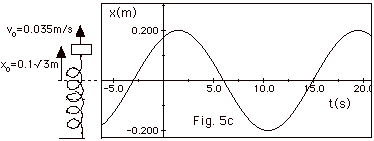
You can work backwards to find t if you know x at that
time.
For example, find t when x = 0.10 m.
Then 0.10 m = 0.20 m cos (0.350 s-1 t-
p/6), or
1/2 = cos (0.350 s-1 t-
p/6).
cos 60o = cos p/3
= 1/2, so
p/3 = (0.350 s-1
t - p/6)
or
0.350 s-1 t = p/3
+p/6 = p/2.
t =(p/2)/(0.350 s-1)
= 4.49 s.
Check Fig. 5 c above for verification of t.
- Sample problems in 107
Problem Set for Simple Harmonic Motion: 10,
14-16, 22-24.
- Examples of simple harmonic motion other than mass-spring
- The definitions of A, T, f, and w
are the same
- The equation of motion when maximum positive displacement
occurs for t = 0 has the same form as x(t) = A cos (wt
+ F) for example, if the
motion is along an arc, the equation could be Q(t)
= Qmax cos
(wt + F)
- For different set-ups, w
is not the same.
- For pendulum, with L = length of pendulum,
d2x/dt2 + (g/L)x = 0
- For mass-spring equation,
d2x/dt2 + (k/m)x = 0
For mass spring, w=
(k/m)1/2.
You see for a pendulum w=
(g/L)1/2 = 2pf
= 2p/T.
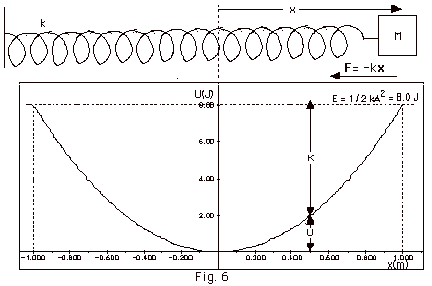
- For a spring with F = -kx,
- The potential energy function U = 1/2 kx2
- Total Energy E = U + K
- For any value of x, E = 1/2 kx2 + 1/2 mv2
For x = ±A, v = 0, because the mass stops momentarily
at both ends. At x = ±A, E = 1/2 kx2
+ 1/2 mv2 becomes:
- E = 1/2 kA2 + 0 = 1/2 kA2
- Figure 6 below shows a plot of U as a function of x. The
total energy
E = 1/2 kA2 = constant. E = U + K, as shown
in Fig. 6. U is greatest at
x = ±A and K is greatest at x = 0.
- Simple Harmonic Motion for Angular Motion
Statement of the problem: Uniform rod of length L is suspended
at a point P. Find the period of the oscillation for small angular
displacements Q.
(See Fig. 7 below)
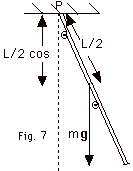
Approach
1: The magnitude of the torque about P due to mg
acting at the center of the rod is (L/2)mg sin Q.
As the rod swings counterclockwise, the torque tends to return
the rod to the equilibrium position, clockwise. The torque then
is -(L/2)mg sin Q. For
small Q, sin Q
≈ Q and the torque =
-[(L/2)mg]Q. Since all the
quantities in the bracket are constants, the torque is directly
proportional to the angular displacement Q
and in the opposite direction. The moment of inertia I of the
rod about one end is 1/3 mL2, or
| tP |
= |
IPa |
| -[(L/2)mg]Q |
= |
(1/3 mL2)d2Q/d/t2 |
| d2Q/dt2
+ (3g/2L)Q |
= |
0 |
Compare this with equation for mass
attached to spring d2x/dt2 + (k/m)x = 0, for which
w = (k/m)1/2, and
see for this case w = (3g/2L)1/2
and T = 2p(2L/3g)1/2.
Approach
2:
The potential energy U of the rod as a function of Q
= mgL(1 - cos Q)/2.
K =1/2 I w2= 1/2
(1/3 mL2) (dQ/dt)2
The total energy = E = mgL(1 - cos Q)/2
+ 1/2 (1/3 mL2) (dQ/dt)2
Since the total energy is a constant,
dE/dt = 0 = mgL sin Q(dQ/dt)/2
+ 1/3 mL2 (dQ/dt)(d2Q/dt2).
For small Q, (mgL/2)Q
+ 1/3 mL2(d2Q/dt2)
or
d2Q/dt2
+ (3g/2L)Q = 0, as before.
Sample problems in 107
Problem Set for Simple Harmonic Motion: 26, 27.
|
|