Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 104

Outline - Center of Mass, Momentum, and Collisions
  1. Linear Momentum p

    1. Linear momentum of an object p = mv, where v is the velocity of an object of mass m. Linear momentum is a vector quantity. p has the same direction as the velocity v.

      1. Newton's second law Fnet external = ma can be rewritten in terms of momentum. Since a = dv/dt, we can rewrite ma = d(mv)/dt. If m is a constant, then you can take it outside of the derivative and m dv/dt goes back to ma. Newton actually wrote the second law as
        Fnet external = dp/dt.

      2. If no net external forces act on a system dp/dt = 0 and the momentum of the system remains a constant. Linear momentum is conserved.

    2. In "collision problems," momentum is always conserved, but energy is only conserved for elastic collisions. In Fig. 1 below, an object of mass m1 = 2 kg moves to the right with a speed of v1i = 5 m/s. It collides with a mass m2 = 3 kg initially at rest.  After the collision, the two objects stick together and move with a velocity vf. We find the velocity after the collision using conservation of momentum.



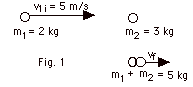
      The initial momentum of the system equals the final momentum:
      pi = pf

      2kg(5 m/s) = (2 + 3)kg vf or vf = 2 m/s

      The initial kinetic energy of the system = 1/2 (2 kg)(5 m/s)2 = 25 J.

      The final kinetic energy of the system = 1/2 (5 kg)(2 m/s)2 = 10 J.

      While momentum is always conserved in a system, kinetic energy is only conserved in an elastic collision. This is an inelastic collision.

    3. Since momentum is a vector quantity, you may consider the conservation of the components of momentum. If two vectors are equal, their components are equal. If the initial momentum pi = pf the final momentum, then:

               (pi)x = (pf)x and (pi)y = (pf)y

      An object of mass 4m is initially at rest. It breaks into three parts, two of mass m and one of mass 2m. After the explosion, one of the objects of mass m moves along the negative Y-axis with a speed of 10 m/s and the other one of mass m moves along the positive X-axes. (Fig. 2a below). Find the magnitude and the direction of the velocity of 2m after the explosion.


      The initial momentum of the system is zero. After the collision one of the pieces moves in the +X direction and the other moves in the -Y direction. To solve this problem you must separate it into two one-dimensional problems.

      (pi)x = (pf)x
      0 = m(10 m/s) + 2mvx
      vx = - 5 m/s  (the minus sign indicates that it is to the left)

      (pi)y = (pf)y
       0 = m(-10 m/s) + 2mvy
      vy = +5 m/s  (the plus sign indicates that it is up)

      v = (vx2 + vy2)1/2 = (25 + 25)1/2 m/s = 5√2 m/s.
      Tangent of the angle that v makes with X axis = vy/vx = 5/-5 = -1.
      Angle Θ with the X-axis = 135o

    4. Kinetic energy is conserved in an elastic collision.



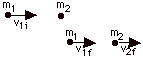
      For the elastic collision shown in the above figure:

      pi = pf

      m1v1i = m1v1f + m2v2f

      Ki = Kf

      1/2 m1v21i = 1/2 m1v21f + 1/2 m2v22f


      Solving Eq. 1 and Eq. 2 for v1f and vf2 (if they are not in the direction shown in the figure, your calculation will give you a minus sign):

      v1f = [(m1 - m2)/(m1 + m2)]v1i v2f = [(2m1)/(m1 + m2)]v1i

      Special cases:

      1. If m1 = m2,  v1f = 0  and  v2f = v1i.
        All momentum and energy of m1 are transferred to m2.

      2. If m2 >> m1,  v1f ≈ - v1i  and  v2f ≈ (2m1/m2)v1i.

        Momentum transferred to m2 = m2v2f = 2m1v1i = 2p1
         for greatest amount of momentum transferred.

        Kinetic energy transferred to m2 = 1/2 m2[(2m1/m2)v1i]2,
        a very small amount, because m1 << m2.

      3. If m1 >> m2,  v1f ≈ v1i.

    5. Sample Problems in 104 Problem Set for Momentum: 5-7, 9-11, 13-23.


  2. Impulse J

    1. Impulse J = d(mv) =dp = Favdt, where Fav is the average force.

    2. Impulse-momentum theorem states that the impulse equals the change in linear momentum. J = mvf - mvi. Since impulse and momentum are vector quantities, you may set the X-component of the impulse equal to the change in the X-component of momentum and the Y-component of the impulse equal to the change in the Y-component of momentum.

    3. Sample Problems in 104 Problem Set for Momentum: 1, 3.



  3. Center of Mass

    1. The center of mass of an extended system is the point whose dynamics typifies the system as a whole when it is treated as a particle.

    2. Sample Problem. Find the location of the center of mass of the system of three particles shown in Fig. 3 below. Each particle has the same mass m. The coordinates (x,y,z) of particle 1 are (0,L,0) of particle 2 are (0,0,0) and of particle 3 (L,0,0).


    3. XCM = (1/3m)[0(m) + 0(m) + L(m)] = L/3
      YCM = (1/3m)[L(m) + 0(m) + 0(m)] = L/3
      ZCM = (1/3m)[0(m) + 0(m) + 0(m)] = 0

    4. Symmetry arguments show that the center of mass of a uniform rectangular plate, a uniform spherical shell, a uniform sphere or a sphere with a spherically symmetric distribution of mass is located at the geometric center of the object.

    5. Sample Problems in 104 Problem Set for Momentum: 2, 4, and 8.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
September 25, 2002
April 17, 2003