Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 108

Outline - Electric Fields
  1. Difference between Action-at-a-Distance and Field Approach

    1. Action-at-a-Distance

      1. Assume one charge acts through a distance to exert a force on another charge.  (Fig. 1 below)



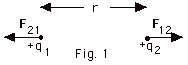
      2. The force F21 of q2 on q1 is equal in magnitude to
        the force F12 of q1 on q2,  but opposite in direction.

        Fe = F21 = F12 = q1q2/4πεor2 = kq1q2/r2
        where 1/4πεo = k = 9.0 x 109 N-m2/C2.

    2. Electric Field

      1. Assume distribution of charge sets up electric field E at point P
        (Fig. 2a below).



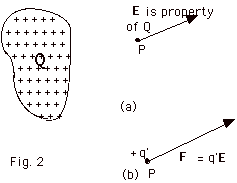
      2. A positive test charge q’ experiences a force Fe = q’E
         (Fig. 2b above)

      3. The magnitude of E is given by E = Fe/q’

      4. The direction of the electric field is that in which a positive test charge is urged.

      5. The field is the property of charges setting up the field.

      6. The electric force Fe is the response of q’ to the electric field.

      7. The principle of superposition holds: If an object is acted upon by two separate influences, it responds to each without modifying its response to the other.


  2. Calculation of Electric Fields

    1. Field due to a point charge +Q at a distance r  (Fig. 3)



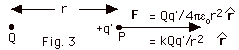
      1. E = Fe/q’ = (kq’Q/r2)/q’ = kQ/r2



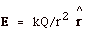
      2. Notice that the electric field depends on the magnitude of Q and the distance r from it, but not on the test charge q’.


    2. Field due to two charges  (Fig. 4)



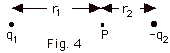
      Field at P due to q1 = kq1/r12 to the right.
      Field at P due to -q2 = kq2/r22 to the right.

      Notice I did not put in the minus sign for field due to q2. I asked what direction a positive test charge placed at P would be urged by the field due to –q2 and saw that was to the right.

      Resultant field at P is kq1/r12 + kq2/r22 to the right.

      When finding a field due to one or more charges you are not asking about a force on the charge or charges that set up the field. Think of the charges setting up the field as set in concrete and assume they are fixed in position.

    3. Distribution of charges

      Although charge is discrete, on a macroscopic level we can consider that the charge is a continuous distribution.

      1. Element of charge dq on length dx is dq = λdx,  where λ is the charge per unit length.

      2. Element of charge dq on area dA is dq = σdA,  where σ is the charge per unit area.

      3. Element of charge dq on volume dV is dq = ρdV,  where ρ is the charge per unit volume.

      4. Apply principle of superposition. Use symmetry principle to eliminate as many components as possible. Remember that integration is not a vector addition.

    4. Examples

      1. Line of charge  (Fig. 5)



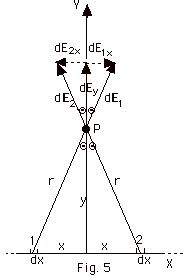
        1. Select symmetrically disposed elements of length dx and charge dq = λdx, where λ is the charge per unit length. The field due to each element at point P,  dE1 = dE2 = kdq/r2.

        2. As shown in Fig. 5,  the X components of dE2x and dE1x cancel.

        3. You are left with the Y components dE1 cos Θ = dE2 cos Θ
          = dE cos Θ= (kdq/r2) cos Θ = k( λ dx) cos Θ/r2.

        4. The variables are x, r, and Θ, while y is a constant. To integrate you must get the expression for dE cos Θin terms of one variable.

        5. Since x = y tan Θ,  dx = y sec2ΘdΘ.
          Also r = y sec Θ   or   r2 = y2sec2 Θ   and
          E = k λ (dx) cos Θ/r2
              = k λ(y sec2ΘdΘ)cosΘ/(y2sec2 Θ)
             = k λ cos ΘdΘ/y.

          E = (k λ/y) ∫cos Θ dΘ

          The final answer depends on the limits.  For an infinitely long line of charge,
          Θ goes from  -π/2  to  + π/2  and
          E = 2k λ/r = λ/2πεor.

      2. The electric field at the center of a semicircle loop of radius R carrying uniform charge Q distributed uniformly over its length  (Fig. 6).



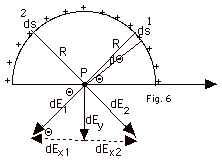
        1. Take symmetrically distributed elements 1 and 2 of length ds and charge dq = λds.  Since dΘ = ds/R in radians, dq = λRdΘ.

        2. dE1 = dE2 = kdq/R2 = dE.
          The directions are as shown in Fig. 6.
          The X-components of dE1 and dE2 cancel.
          dEy = dE sin Θ = (kdq/R2) sin Θ = k λRdΘsin Θ/R2 = k λdΘsin Θ/R.

        3. E = 

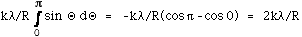
        4. Since λ= charge/length = Q/ πR,
          E = 2k(Q/ πR)R = 2(1/4πεo)(Q/ πR2)= Q/2 π2 εoR2.
          E = - Q/2 π2 εoR2 j.

      3. Electric Field due to a Uniformly Charged Disk  (Fig. 7)



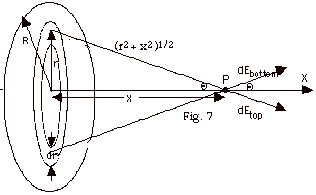
        Divide the disk into rings of radius r and thickness dr. If you cut the ring and pull it out into a rectangle, it has length 2 πr and thickness dr. Its area dA = 2 πr dr. If its charge per unit area = dq/dA = σ, dq = σdA = σ2 πr dr. The field due to a small amount of charge dq at the top of the ring of radius r is dEtop = kdq/(x2 + r2) down and to the right as shown in Fig. 7.  The electric field for an equal amount of charge dq from the bottom is dEbottom = kdq/(x2 + r2) up and to the right as shown in Fig. 7.  The dE's from the entire ring form a cone about point P.  The components in the Y and Z direction cancel, leaving the resultant field in the +X - direction.

        The component of the dE in the X-direction is:
        kdq/(x2 + r2) cos Θ.
        From Fig. 7, we see that cos Θ = x/(x2 + r2)1/2,    so
        dEx = kdq/(x2 + r2) cos Θ = kdqx/(x2 + r2)3/2.
        For the ring of Fig. 7 with charge  σ2 πr dr,

        dE = k(σ2 πr)xdr/(x2 + r2)3/2



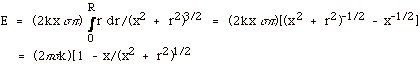
  3. Electric Flux  ΦE
    1. Definition



      dA is an element of area.
      The direction of dA is that of the outward normal to the surface.


    2. Examples




  4. Gauss's Theorem

    1. .

    2. ΦE  is the total flux crossing a three dimensional surface.

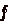    3. is the integral around a closed three dimensional surface.

    4. Application

      Choose Gaussian surface that gives maximum symmetry properties such that:

      1. E is normal to the surface, that is, the angle between E and dA is zero and E . dA = E dA cos 0o = E dA.

      2. E is constant everywhere on the surface, that is, .


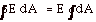    5. Examples

      1. Spherically symmetrical distribution of charge  (Fig. 10)




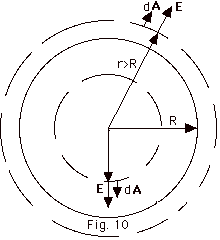
        1. For hypothetical Gaussian surfaces (shown by dashed cross-section of spheres in figure above), choose sphere.  E must be normal to surface and constant at any point on a Gaussian surface or the symmetry of a sphere would be unraveled by rotating it.

          For  r > R  or  r < R,   E, dA must be zero and E is constant everywhere on the Gaussian surface.



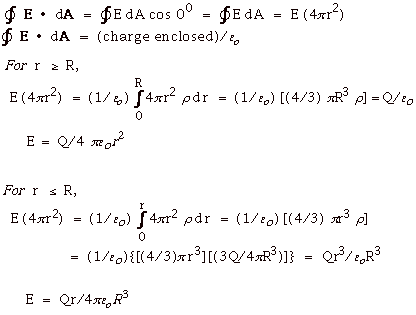
        3. Continuity Conditions

          For  r ≤ R  at  r = R,   E = Q/4πεoR2

           For  r ≥ R  at  r = R,   E = Q/4πεoR2


      2. Field of a cylinder  (Fig. 11)




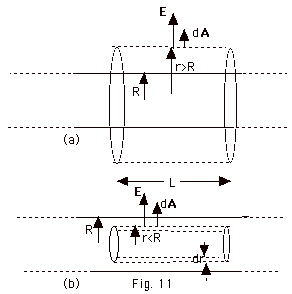
        1. For hypothetical Gaussian surface choose cylinder coaxial with the real cylinder.  E must be normal to the surface and constant at any point on cylinder or symmetry of cylinder would be unraveled by rotating it.

          For  r < R  or  r > R,   E, dA = 0 and E is constant everywhere on the Gaussian surface,   so

        2.  


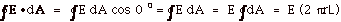
          For  r > R,  charge enclosed = (charge/length)L = λL,
          E(2 πrL) = λL   or   E = λ/2πεor              (Fig. 11 a)

          For  r < R,  charge enclosed = (charge/volume)(volume) = ρ( πr2L),
          E(2 πrL) = ρ( πr2L)/ εo   or   E = ρr/2 εo           (Fig. 11 b)


        3. Continuity conditions

          For  r ≥ R  at  r = R,   E = λ/2πεoR

          For  r ≤ R  at  r = R,   E = ρR/2 εo = [Q/( πR2L)]R/2 εo
                                                              = (Q/L)/2πεoR
                                                             = λ/2πεoR


      3. Field of an infinite flat sheet of charge  (Fig. 12)



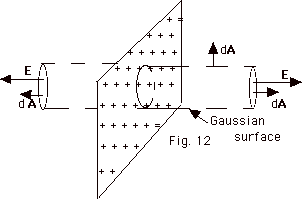
        1. For a hypothetical Gaussian surface choose a beer can with ends parallel to the sheet.

        2. Electric field must be perpendicular to sheet and independent of distance from sheet to preserve symmetry property of an infinite plane.

          For end caps of beer can,  E, dA = 0.
          For sides of beer can,  E, dA = 90o.

        3. For end caps of beer can, taking into consideration both ends,



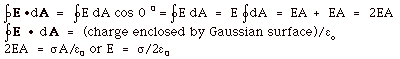
          For sides of beer can, E dA cos 90o = 0.


  5. Conductors

    1. The definition of a conductor is that electrons are perfectly free to move in it.  If they are free to move, they will move until there is no electric field in the conductor.

    2. By Gauss's law, .
      If there is no electric field in the conductor, then a Gaussian surface very near the surface of the conductor contains no charge.  The charge must be on the outside of the conductor.



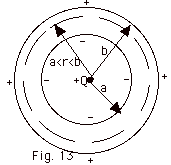
    3. Figure 13 above shows the cross-section of a spherical shell of inner radius a and outer radius b.  At the center of the shell is a point charge +Q.  The Gaussian surface of radius r such that  a < r < b  is inside the conductor so there can no be field there.  The +Q charge attracts electrons so that the charge on the inside of the conducting shell is –Q,  leaving the outside of the shell positively charged with +Q.  While the charge on the surface of radius a is the same as the surface of radius b, the charge per unit area on each is not the same.

      σb = +Q/2 πb2  and   σa = - Q/2 πa2


  6. Electric Field of a Dipole

    1. Description

      1. Dipole consists of two point charges of opposite sign, but equivalent quantity of charge.

      2. The charges are separated by a distance d = 2a  in Fig. 14 below.



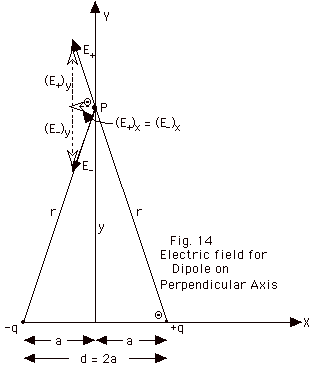
      3. We are often interested in cases for which the distance from the center of the dipole to the point P where we wish to find the electric field is much greater than d the distance of separation of the charges.

    2. Calculation of E for point on perpendicular bisector (Fig. 14)

      1. At P,  the electric field due to the positive charge is
        E+ = kq/r2 = kq/(a2 + y2)
        upward along the line connecting +q and P.

      2. At P,  the electric field due to the negative charge is
        E+ = kq/r2 = kq/(a2 + y2)
        downward along the line connecting - q and P.

      3. Notice that the magnitudes of the field due to +q and - q are equal. In Fig. 14, we see the Y-components of the electric field due to +q and the electric field due to -q,  (E+)y and (E2)y , respectively, cancel.

      4. The components of the electric field in the X-direction,
        (E+)x = E1 cos Θ and (E2)x = E2 cos Θ, are equal because E1 = E2. These components are in the same direction, so they add. The total electric field at P due to both charges  E = 2[kq/(a2 + y2)] cos Θ.  From the figure, we see that cos Θ = a/(a2 + y2)1/2,  so E = k(2qa)/(a2 + y2)3/2.

      5. For  y >> a,  E = k(2qa)/y3 = k(qd)/y3 = kp/y3,  where p is called the dipole moment. We can make p a vector quantity by taking its direction from the minus charge to the positive charge. The direction of the electric field in Fig. 14  is in the negative X-direction, so we may write for  y >> a,  E = - kpi/y3.


    3. Calculation for electric field on axis of dipole (Fig. 15)



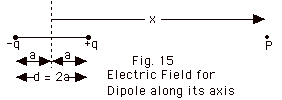
      1. Electric field at P due to - q is kq/(x + a)2  to the left.

      2. Electric field at P due to +q is kq/(x - a)2  to the right.

      3. Taking to the right to be positive, the total electric field at P due to both charges is
        E = kq[1/(x - a)2 - 1/(x + a)2]
           = kq{(x + a)2 - (x - a)2}/(x2 - a2)2
           = kq{x2 +2ax + a2 - x2 +2ax - a2}}/(x2 - a2)2
           = k4aqx/(x2 - a2)2 = 2kpx/(x2 - a2)2
        For  x >> a,  E = 2kp/x3  and  E = 2kpi/x3.

        Again for great distances,  E is inversely proportional to the cube of the distance from the center of the dipole.


  7. Two Problems Concerning Electric Fields

    1. One problem is, given a distribution of electric charges, find the field due to them. For non-symmetrical distributions, we have used a vector addition of the field due to each charge or element of charge. For symmetrical distributions, we have used Gauss's theorem.

    2. The other problem is, given an electric field E, find the force on a charge particle in the field. Since the electric field is the force per unit charge, Fe = qE.  If the charge is positive, the force will be in the same direction of the field. If the charge is negative, the force will be opposite to the direction of the field.


  8. Torque on a dipole in an Electric Field

    1. Dipole moment  p = qd. The direction of p is from the minus charge to the plus charge.

    2. In a constant electric field, the force on the positive charge F+ = qE to the right and the force on the negative charge F- = qE to the left, as illustrated in Fig. 16 below.



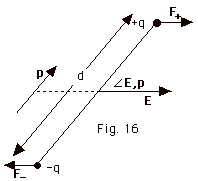
    3. The torque due to F+ about an axis through a point half way between the charges is (qEd/2) sin E, p into the page. The torque due to F- is also (qEd/2) sin E, p into the page. The total torque = qdE sin E, p into the page. A convenient way of writing the torque is τ = p x E.


  9. Sample Problems from 108 Problem Set for Electric Fields: 1-8, 10, 14-17, 19-27.




Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
August 8, 2002
April 29, 2003