Click here to return to Phyllis Fleming's homepage Phyllis Fleming Physics

Physics 108

Outline - Physical Optics
  1. Descriptive Terms of Wave Motion

    1. Amplitude is the maximum displacement from the equilibrium position.

    2. Velocity is the distance moved by the wave in one second. The velocity of a wave depends on the medium in which the wave travels.

    3. Wavelength λ is the distance moved by the wave in one complete oscillation of the source. The distance between crests is one wavelength. The wavelength depends on the medium in which the wave travels.

    4. Frequency f is the number of oscillations of the source per second. Frequency depends on source and does not change when wave goes into a different medium.

    5. Period T is the time for one complete oscillation. The period depends on the source and does not change when the wave enters a different medium.

    6. Velocity v is the distance moved by the wave in one second.

    7. (distance moved/oscillation)(#of oscillation/s) = (distance/s)
      ( λ)(f) = v


  2. Light in a medium

    1. The index of refraction n of a medium is defined as the ratio of the speed of light in a vacuum (or air) c to the speed of light in a medium v.
      n = c/v

    2. In a vacuum (or air), for light of frequency f, wavelength λo,
      and velocity c,
      λof = c                  (Equation 1)
    3. In a medium of index of refraction n, for light of frequency f,
      wavelength λn, and velocity v,
      λnf = v                 (Equation 2)
      Dividing Eq. 1 by Eq. 2,
      λo/ λn = c/v = n  or  λn= λo/n.
      Wavelength is directly proportional to velocity. When the velocity of a wave in a medium decreases the wavelength decreases.


  3. Interference

    1. Constructive and Destructive interference

      1. Constructive interference occurs when a crest falls on a crest and a trough falls on a trough. Destructive interference occurs when a crest falls on a trough.

      2. For constructive interference, the path difference must equal an integral number of wavelengths. For destructive interference, the path difference must equal half-integral number of wavelengths.

      3. In Fig. 1 below, the wave from source 1 has traveled a distance S1P to point P and the wave from source 2 has traveled a distance S2P to point P. The path difference = S1P - S2P.



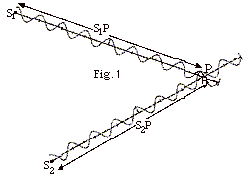
      4. For constructive interference, S1P - S2P = m λ, m = 0, 1, 2, etc.

      5. For destructive interference, S1P - S2P = (m + 1/2) λ.

    2. Fig. 2 below shows two point sources, S1 and S2, emitting waves that are detected at a distant point P from the two sources. The distances r1 and r2 from sources 1 and 2 to the point P are large compared to the separation d of the sources. For this case, the two rays along the lines of sight from the two sources to point P are nearly parallel, both being essentially at the same angle Θ from the X axis as shown in the figure. Since I am unable to draw such large distances and at the same time show the path difference, S1P - S2P = S1B, as a reasonable length, I have made a “break” in the drawing as the two "parallel" rays come together and arrive at P.



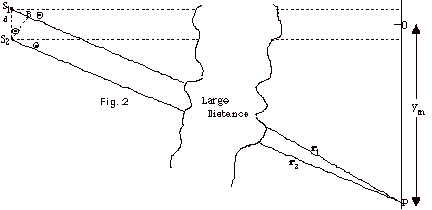
      1. If point P is one of maximum intensity (constructive interference),
        S1B = m λ.
        If point P is one of minimum intensity (destructive interference),
        S1B = (m + 1/2) λ.

      2. From triangle BS1S2, we see that sin Θ = S1B/d  or  d sin Θ = S1B.

        1. For constructive interference, d sin Θ = m λ.

        2. For destructive interference, d sin Θ = (m + 1/2) λ.

    3. In Fig. 3 below, ym represents the distance on the screen from center of the intensity pattern to a position of constructive or destructive interference and L the distance from the slits to the screen.

      sin Θ = ym /(L + ym2)1/2



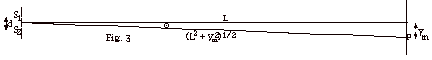
      1. For ym << L,  sin Θ is approximately = ym/L = tan Θ

      2. When ym << L

        1. For constructive interference,

          1. sin Θ = m λ/d = ym/L  or  ym = m λL/d

          2. ym + 1 – ym = [(m + 1) – m] λL/d = λL/d =
            distance between maxima.

        2. For destructive interference,

          1. sin Θ = (m + 1/2) λ/d = ym/L  or  ym = (m + 1/2) λL/d

          2. ym + 1 – ym = [(m + 1 + 1/2) – (m + 1/2)] λL/d = λL/d = distance between minima.

    4. Sample Problems: 108 Problem Set for Physical Optics, 1-5, 12, 15, 16, and 17.


  4. Phase Change at Interface




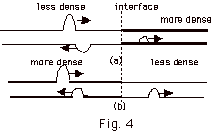
    1. When a pulse goes from a less to a denser medium, the reflected wave experiences an 180o or π phase change. When it goes from a more to a less dense medium, there is no change of phase for the reflected wave. (Fig. 4 above).

      From a crest to a trough is a distance of λ/2. A change of phase of 180o or π is equivalent to a path difference of λ/2. Notice that there is never a change of phase for the transmitted ray at an interface. The speed of light is greatest in a vacuum or approximately air with index of refraction n = 1 = c/v. The higher the index of refraction, the smaller the speed of the wave.

      When light goes from medium 1 to medium 2, the reflected ray will experience a phase change of   π  if  n2 > n1.  There will be no phase change if  n2 < n1.

    2. Boundary Conditions Applied to Waves



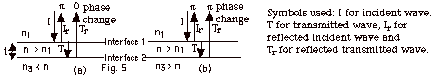
      1. In Fig. 5a above, the incident wave I is reflected at the first interface of a less dense-more dense medium, where the index of refraction in the incident medium  n1 < n,  the index of refraction in the film. There is an 180o or π change in phase at the first interface. There is no change in phase for the transmitted wave T. And there is no change in phase at the second interface of a more dense-less dense medium with  n3 < n.

        The "real" path difference for 5a or 5b above is 2t, where t is the thickness of the medium. A change of phase of π for the incident ray is equivalent to an additional path difference of λn/2, where λn is the wavelength in the film. For Fig. 5, the index of refraction of the film is n. Remember that the wavelength in a medium λn = λ/n where λ is the wavelength in a vacuum.

        The condition for

        1. maximum:
          2d = (m +1/2) λn   or
          2d = (m +1/2) λ/n   or
          2nd = (m +1/2) λ
        2. minimum:
          2nd = m λ
        3. Because of the change in phase of π, the conditions for maxima and minima have been reversed.

      2. In Fig. 5b, the incident wave I is reflected at the first interface of a less dense-more dense medium where the index of refraction in the incident medium n1 < n,  the index of refraction in the film. There is an 180o or π change in phase at the first interface. There is no change in phase for the transmitted wave T. There is another change of phase of π at the second interface since n3 > n. The total phase change is 360o or 2 π. That is equivalent to no phase change overall.

        The condition for

        1. maximum:
          2nd = m λ
        2. minimum:
          2nd = (m + 1/2) λ
    3. Sample Problems: 108 Problem Set for Physical Optics,  13, 14, 19 and 20.


  5. Interference for Multiple Slits

    1. For multiple slits with equal spacing d, the condition for maxima is identical to that for 2 slits with a separation of d:  sin Θ = m λ/d

    2. Rather than giving the spacing d, you usually get the number of slits per length. The reciprocal of the number of slits per length is equivalent to d, but be careful that the length is in meters.

    3. Sample Problems: 108 Problem Set for Physical Optics,  12, 16, and 26.


  6. Diffraction (Intensity pattern shown in Fig. 8 below)



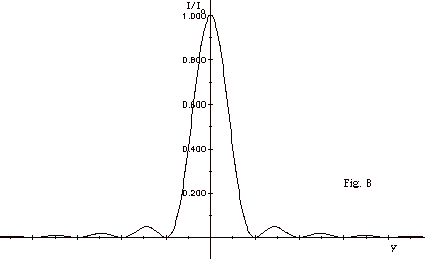
    1. Diffraction is really interference from a very large number of sources and slits.

    2. Think of a single slit as made up of an infinite number of sources and slits. In Fig. 7 below, I have only divided it up into a few sources.



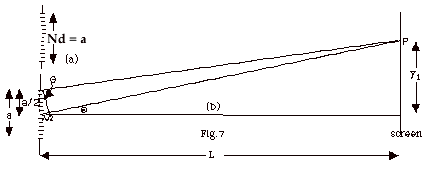
    3. In Fig. 7a, with N the number of slits and d the distance between each slit, the slit width a = Nd.

    4. Conditions for a Maximum

      1. Since the condition for a maximum is the same for N slits with a separation d as it is for 2 slits with a separation d, you get a maximum when sin Θ= m λ/d = Nm λ/a.

      2. Since N is a very large number, sin Θ will be greater than 1 unless m = 0. There is only one principal maximum and that occurs at the center of the screen.

    5. Conditions for a Minimum in a Diffraction Pattern

      1. The width of the slit is a. Think of light coming from an element of length dy at the top of the slit and an element of length dy half way down on the slit as shown in Fig. 7b above. Obviously there is a path difference between them when the light from the top and middle of the slit arrive at P.

        1. Destructive interference occurs if this path difference for the element at the top of the slit and the element at the center of the slit is λ/2. This will also be true for other elements that you pair as you go down the slit with path differences of λ/2.

        2. In the little triangle on the left, for destructive interference, sin Θ = ( λ/2)/(a/2) = λ/a, the condition for the first minimum.

      2. For the second minimum, divide the slit into four parts and match elements that give a path difference of λ/2. The second minimum occurs for sin Θ = ( λ/2)/(a/4) = 2 λ/a.

      3. Continuing in this way, you find for the mth minimum:
        sin Θ = m λ/a
    6. Position of Minima on Screen

      1. From Fig. 7 above,  tan Θ= y/L.   For small Θ,  tan Θ ≈ sin Θ.

      2. From VI, E, 3 above, sin Θ = m λ/a.
        For sin Θ ≈ tan Θ,   m λ/a = ym/L,   or
        ym = m λL/a,  where ym corresponds to the mth mimimum.

      3. The distance between minima =
        Δy = ym+1 - ym = (m + 1) λL/a - m λL/a = λL/a.

      4. Since the width of the central maximum = the distance between the first minimum on the right and the first minimum on the left of the central maximum, the width of the central maximum = 2 λL/a.

    7. Sample Problems: 108 Problem Set for Physical Optics,  21, 22, and 24.


  7. Diffraction Limit

    1. Diffraction poses a fundamental limit on the ability of optical systems to distinguish closely spaced objects.

      1. Assume light from two point sources far from a slit illuminates the slit. Light diffracting at the slit produces two single-slit diffraction patterns, one for each source as shown in Fig. 9 below.



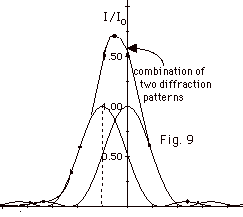
      2. Because the sources are at different angular positions, the central maxima of the diffraction patterns overlap.

      3. The result in Fig. 9 is one big blob and you can't distinguish two separate objects.

    2. Rayleigh's Criterion

      1. If the angular separation of the two objects is great enough, you see a separation of the two maxima and can distinguish the existence of two objects.

      2. The two peaks are barely distinguishable if the central maximum of one coincides with the first minimum of the other. We found earlier that sin Θ = λ/a for the first minimum in single-slit diffraction. The angular separation between the diffraction peaks equals the angular separation between the sources. In most optical systems, the wavelength of the light is much smaller than the aperture. In the small angle approximation, sin Θ is approximately equal to Θ, the Rayleigh condition that the two sources be just resolvable gives for a slit  
        Θmin = λ/a
      3. The Rayleigh criterion for circular apertures is                                  
        Θmin = 1.22 λ/D,
        where D is the aperture diameter.

    3. Sample Problem: 108 Problem Set for Physical Optics,  25.


  8. Polarization

    1. Polarization is the attribute that a wave's oscillation have a definite direction relative to the direction of propagation of the wave.

      1. The direction of the polarization is parallel to the electric field of the electromagnetic wave and perpendicular to the direction of the propagation of the wave.

      2. Light waves are transverse waves that may be polarized.

    2. Natural light is partially polarized. This means that the electric field vectors associated with this light are not in one direction.

      1. The electric field vectors can be resolved into two vectors perpendicular to each other.

      2. When you send light through a polaroid, the electric field vector perpendicular to the transmission axis is absorbed, leaving only the component along the transmission axis of the polaroid.

      3. When unpolarized light with intensity of the incident light equal
        to Ii is sent through a polaroid, the intensity of the light is reduced by a factor of 2. The transmitted intensity It = Ii/2.

    3. Polarized Light passing through a polaroid

      1. Figure 13a below represents polarized light with incident electric field Ei approaching a polaroid. The direction of the transmission axis is indicated with a dash line. The transmitted electric field Et is smaller and rotated through an angle Θ along the transmission axis.



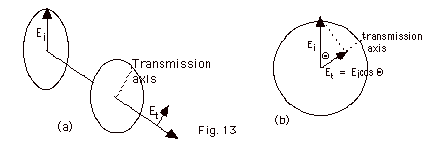
      2. Figure 13b above gives an end view. The component of E1 along the transmission axis is the transmitted electric field Et = Ei cos  Θ.

      3. The Poynting Vector = the intensity of the light I = Emax2/2µoc.
        The intensity is proportional to the electric field, that is,
        I = kE2, where k is a constant.

        1. The initial intensity Ii = kEi2

        2. The transmitted intensity It = kEt2 = kEi2 cos2 Θ

        3. It/ Ii = cos2 Θ

    4. Polarization by Reflection

      1. The incident ray in Fig. 15 below is unpolarized. It has components of the electric field perpendicular to the plane of the paper represented by a dot • and a parallel component in the plane of the paper represented by an arrow .



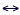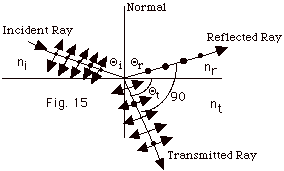
      2. When the angle between the reflected ray and the transmitted ray is 90o, the reflected ray cannot contain any of the parallel component because then the electric field would be in the direction of the propagation and that is not possible. The transmitted ray has parallel components of the electric field and some perpendicular components of the electric field.

      3. When the reflected ray has only components of the electric field perpendicular to the paper, the reflected light is plane polarized. The angle of incidence when you get plane polarized light in the reflected ray is called the Brewster angle ΘB.

        1. Snell's law states that ni sin Θi = nt sin Θt.

        2. For Θi = ΘB,  Θr + Θt = 90o.

        3. Since Θi = Θr,  and for this case Θi = ΘB,
          ΘB + Θt = 90o  or  Θt = 90o - ΘB

        4. Snell's law becomes ni sin ΘB = nt sin (90o - ΘB) = nt cos ΘB  or
          tan ΘB = nt/ni.

        5. If the light is incident from air with nI = 1,
          tan ΘB = nt.

    5. Sample Problems: 108 Problem Set for Physical Optics,  28 - 31.


  9. Addition of Wave Amplitudes Using Phasors

    1. Imagine we have two waves with different amplitudes.  We can write the equations of the waves
      y1(r1,t) = A1 cos (ωt - kr1)   and
      y2(r2,t) = A1 cos (ωt - kr2),
      where ω = 2 πf and f the frequency of the waves,  and
      where k = 2 π/ λ and λ the wavelength of the waves.

      We can represent them as the horizontal components of a vector that rotates counterclockwise with angular speed ω.

      The length of the vector represents the amplitude of the wave and the angle between them represents the phase difference ΔΦ, as illustrated in Fig. 16 below.



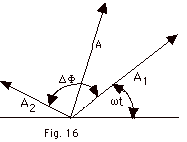
      The phase angle is defined as (2 π/ λ)(r2 - r1), where (r2 - r1) is the path difference. The resultant amplitude is the horizontal component of the vector sum.

      1. If  ΔΦ = 2m π  for m = 0, 1, 2, . . .
        as in Fig. 17 a below, the interference is constructive.



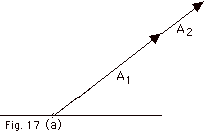
        For ΔΦ = 2m π, the path difference (r2 - r1) = m λ as we found before.


      2. If  ΔΦ = 2 π (m +1/2)  for m = 0, 1, 2, . . .
        as in Fig. 17 b below, the interference is destructive.



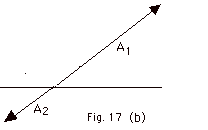
        For ΔΦ = 2m π, the path difference (r2 - r1) = (m + 1/2) λ as we found before.

    2. Using non-rotating phasor diagrams for determining maximum amplitude at a point

      Example:

      Two radio transmitters both of the same initial phase and amplitude have a wavelength of 30 m. A distance of 25 m separates the transmitters. We wish to find the resultant amplitude of these two radio transmitters in the directions (a), (b) and (c) shown in Fig. 18 below.



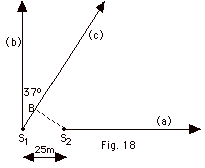
      1. For direction (a) the path difference is 25 m and
        ΔΦ= (2 π/ λ)(r2 - r1) = (2 π/30 m)(25 m) = 5 π/3 = 300o.

        The phasor diagram for this direction is shown in Fig. 19a below.



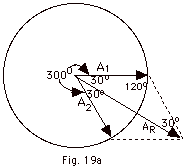
        Since the amplitude of both transmitters is the same, we draw a circle for the phasor diagram. We arbitrarily draw A1 to the right on what would be the X-axis and A2 rotated counterclockwise at an angle of 300o. We draw the diagonal of the parallelogram to find the resultant amplitude AR. By geometric considerations, we see the small angles of the triangle are 30o, leaving the angle opposite the resultant amplitude as 120o.

        Letting A1 = A2 = A  and using the law of cosines,
        AR2 = A2 + A2 - 2A2 cos 120o = 3A2.

        Since the intensity of a wave is proportional to the square of the amplitude, the resultant intensity is three times the intensity of either source.

      2. For direction (b) the path difference equals zero (the parallel rays meet at a very far distance away). The resultant amplitude is 2A and the resultant intensity is proportional to 4A2.

        The phasor diagram for this direction is shown in Fig. 19b below.



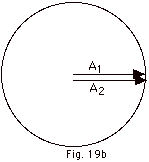
        The intensity is four times the intensity due to one source.


      3. For direction (c) the path difference = the length S1B in Fig. 18.

        This path difference = 25 m sin 37o = 15 m.
        ΔΦ= (2 π/ λ)(r2 - r1) = (2 π/30 m)(15 m) = π = 180o.

        The phasor diagram for this direction is shown in Fig. 19c below.



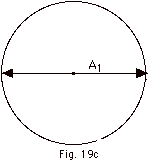
        The resultant intensity is zero.


    3. Sample Problems: 108 Problem Set for Physical Optics,  6, 7, and 9.

Homepage Sitemap
     

Website Designed By:
Questions, Comments To:
Date Created:
Date Last Updated:

  

Susan D. Kunk
Phyllis J. Fleming
August 8, 2002
May 2, 2003